Tangent Line to a Curve
Key Questions
-
The "tangent slope" is the slope of the tangent line. It is also called "the slope of the tangent" and "the slope of the curve at a point".
-
Answer:
You could use infinitesimals...
Explanation:
The slope of the tangent line is the instantaneous slope of the curve. So if we increase the value of the argument of a function by an infinitesimal amount, then the resulting change in the value of the function, divided by the infinitesimal will give the slope (modulo taking the standard part by discarding any remaining infinitesimals).
For example, suppose we want to find the tangent to
#f(x)# at#x=2# , where:#f(x) = x^3-3x^2+x+5# Let
#epsilon > 0# be an infinitesimal value. Then:#(f(2+epsilon) - f(2))/epsilon# #=(((2+epsilon)^3-3(2+epsilon)^2+(2+epsilon)+5)-((2)^3-3(2)^2+(2)+5))/epsilon# #=(((8+12epsilon+6epsilon^2+epsilon^3)-3(4+4epsilon+epsilon^2)+(2+epsilon)+5)-(8-12+2+5))/epsilon# #=((12epsilon+6epsilon^2+epsilon^3)-(12epsilon+3epsilon^2)+epsilon)/epsilon# #=(epsilon+3epsilon^2+epsilon^3)/epsilon# #=1+3epsilon+epsilon^2# of which the standard (i.e. finite) part is
#1# (discarding the#3epsilon+epsilon^2# ).So the slope of the tangent is
#1# and the tangent point is:#(2, f(2)) = (2, 3)# So the equation of the tangent may be written:
#(y-3) = 1(x-2)# or more simply:
#y = x+1# graph{ (y-(x^3-3x^2+x+5))(y-x-1) = 0 [-3.355, 6.645, 1.38, 6.38]}
-
The tangent line to a curve at a given point is a straight line that just "touches" the curve at that point.
So if the function is f(x) and if the tangent "touches" its curve at x=c, then the tangent will pass through the point (c,f(c)). The slope of this tangent line is f'(c) ( the derivative of the function f(x) at x=c).
A secant line is one which intersects a curve at two points.
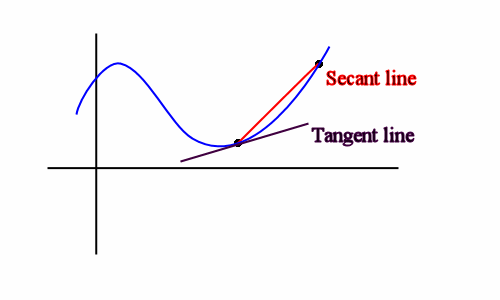
Click this link for a detailed explanation on how calculus uses the properties of these two lines to define the derivative of a function at a point.
Questions
Derivatives
-
Tangent Line to a Curve
-
Normal Line to a Tangent
-
Slope of a Curve at a Point
-
Average Velocity
-
Instantaneous Velocity
-
Limit Definition of Derivative
-
First Principles Example 1: x²
-
First Principles Example 2: x³
-
First Principles Example 3: square root of x
-
Standard Notation and Terminology
-
Differentiable vs. Non-differentiable Functions
-
Rate of Change of a Function
-
Average Rate of Change Over an Interval
-
Instantaneous Rate of Change at a Point