Question

A spring with a constant of `4 (kg)/s^2` is lying on the ground with one end attached to a wall. An object with a mass of `6 kg` and speed of `5 m/s` collides with and compresses the spring until it stops moving. How much will the spring compress?

Answer 1

The spring will be compressed by
`6.12 m`, rounded to second decimal place.

Explanation:

`E_(el)=>`Elastic Energy of compressed or elongated spring.
`E_k=>` Kinetic Energy of object
`m=>`Mass of object
`v=>`Velocity of object
`k=>`Constant of the spring
`x=>`Deformation of the spring

The energy from the object that collides with the spring is kinetic energy. In the process, the kinetic energy will converted to elastic energy of the spring.

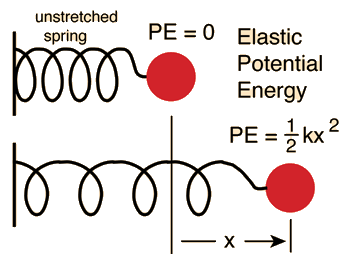
In the given problem the spring is being compressed by the object.

After the object has stopped and the spring has been compressed to the maximum, all the object's kinetic energy has got converted into elastic potential energy of the spring. Equating both energies we obtain

`E_k=E_(el)` ......(1)
We know that kinetic energy of the object `=1/2mv^2` and elastic potential energy of the compressed spring is shown in the picture. Inserting in(1)
`1/2m*v^2 = 1/2k*x^2`
Multiplying both sides with 2, we get

`m*v^2 = k*x^2`
Put our known values in the above equation and solving for `x`
`6 * 5^2 = 4 *x^2`
`x^2 = 150/4`
`x=sqrt((75)/2) = 6.12 m`, rounded to second decimal place.