Question

How do you evaluate sine, cosine, tangent of `pi/4` without using a calculator?

Answer 1

`pi` means 180 °

Explanation:

Hence `pi/4` means `180/4` which is 45 ° .

`45 °` is a special triangle where the measurements are

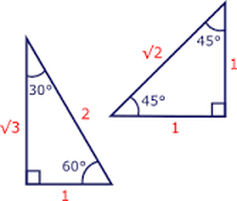
Now, you can find the sine, cosine and tangent without a calculator!

You should obtain

Sin and cosine: `1/(sqrt2)`
Tangent: `1`

I am assuming you are familiar with the formula SOH CAH TOA, yes?

Answer 2

Start by drawing a right triangle in Quadrant I, with a `45^@` angle, which is the equivalent angle for `pi/4`.

Explanation:

The `45^@-45^@-90^@` right triangle has legs of equal length, so the ratio for tangent = 1. (opposite over adjacent)

I teach that `x^2+x^2=1` is the Pythagorean Theorem for the isosceles right triangle with a hypotenuse = 1. To solve, you get `2x^2=1`, so `x^2=1/2`.
Take the square root of both sides:
`x = +-sqrt(2)/2`

If each side = `sqrt(2)/2`, then both the sine and cosine are equal to that value since the hypotenuse is 1.