z^alpha/{z^n+1} has simple poles at
z_k=exp(ipi(1/n+{2k}/n), \quad \quad k=0, 1, 2, ...\quad (n-1)
Note that d/dz[z^n+1]=nz^{n-1} is analytic for n>1 and is not zero at z_k. Therefore, z_k is a zero of order 1 of (z^n+1) which lets us write:
(z^n+1)=(z-z_k)g(z)
where g(z) is analytic and g(z_k)\ne 0. It follows that
z^alpha/{z^n+1}= { z^alpha/g(z)}/{(z-z_k)}
The residue at z_k is
B_k=(z_k)^alpha/g(z_k)
To find g(z_k) use,
d/dz[z^n+1]=d/dz[(z-z_k)g(z)]
nz^{n-1}=g(z)+(z-z_k)g'(z)
Sub z=z_k \quad \implies \quad g(z_k)=n(z_k)^{n-1}
\implies \quad B_k=(z_k)^alpha/{n(z_k)^{n-1}}=1/n(z_k)^{1+alpha-n}
Now we must choose an integration path. The problem is choosing a path that won't have a singularity on it for any value of n. I am going to use the path described below.
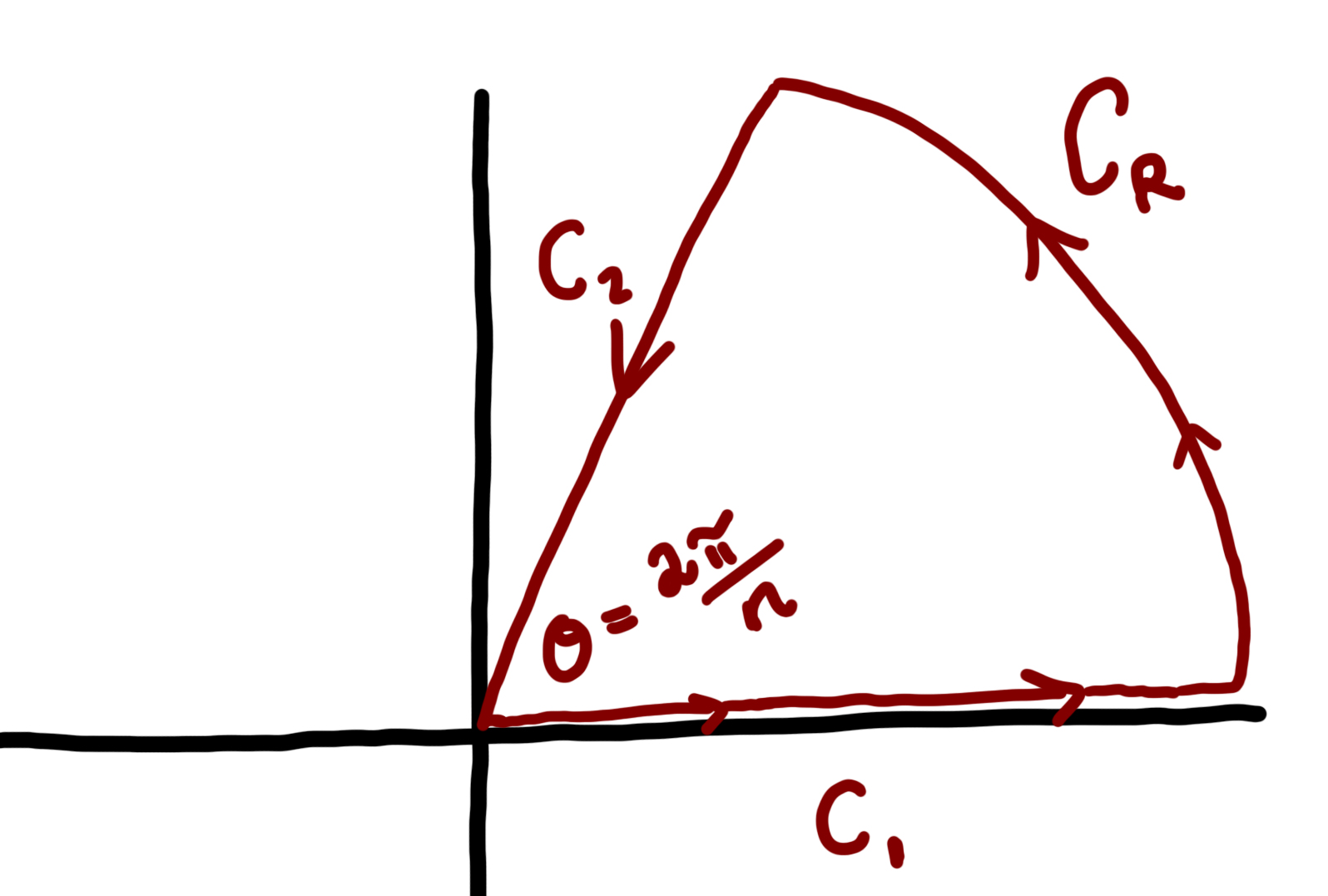
C_1=int_0^inftyx^alpha/(x^n+1)dx
C_2=int_infty^0(Re^{i{2pi}/n})^alpha/((Re^{i{2pi}/n})^n+1)dR=e^{i2pialpha/n} int_infty^0 R^alpha/(R^n+1)dR
Note that
C_2=-e^{i2pialpha/n}C_1
Before evaluating C_R consider
|z^alpha/(z^n+1)| leq R^alpha/{R^n-1}
So |C_R| \leq R^alpha/{R^n-1} 2pi R=2pi 1/{{(R)^{n-(alpha+1)}}-R^{-alpha-1}}
We are given that n>(alpha+1), Therefore lim_{R\rarr infty}C_R=0
Note that I have chosen theta in the diagram so that only one singularity is enclosed. Therefore we only have to consider the first residue, B_0.
C_1+C_2+C_R=2pi i B_0
Substitute values and solve for C_1
C_1(1-e^{i2pialpha/n})+0=2pii1/n(z_0)^{1+alpha-n}
Sub z_0 To get the answer:
int_0^inftyx^alpha/(x^n+1)dx=C_1={2pii}/{n}1/(1-e^{i2pialpha/n})e^{ipi(1+alpha-n)/n}

