Question

How can I work with multiplication factor in unit conversions? I don't understand how they work!

Answer 1

Here's how you can think about that.

Explanation:

I assume that you're having some difficulties with decimal multiples and submultiples for various units.

The metric system is based around powers of 10. In your example, 1 meter is the basic unit of measurement for length.

Here's how the multiplication factors would look like

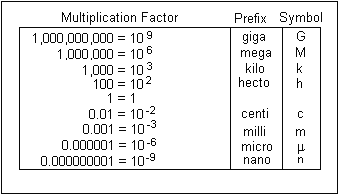
The metric system boils down to using the basic unit, which in this case is the meter, to express a wide variety of lengths.

For exampe, if you measure something to have a length of 100 meters, then you need to multply the basic unit, which is the meter, by a factor of `10^2` to get the length of 100 meters.

So, every time you have 100 meters, you have

`10^2 * "1 meter" = "100 meters" = "1 hectometer" = "1 hm"`

In your example, 1000 km cannot be equal to 1 meter, since to get from one meter, which is the basic unit, to one kilometer, you need to multiply by a factor of `10^3`.

This means that it's actually the other way around

`"1 km" = 10""^3 * "1 meter"`

The same idea applies when you have less than one meter. For example, 1 milimeter can be reached by dividing the basic unit by a factor of `10^2`.

This is exactly the opposite of getting from 1 meter to 1 kilometer. When you measure long distances, you need multiples of the basic unit `->` you multiply by factors of `10`.

When you measure short distances, you need submultiples of the basic unit `->` you divide by factors of `10`.

You need to learn each prefix and its symbol in order to be able to do conversion very easily, but once you understand that everything revolves around how things relate to the basic unit.

Once you learn them, you will have no problem understand that mega is `10^6` bigger than `1`, or that *nano is `10^9` smaller than `1`.