Question

Molecular orbital theory for polyatomic molecules?

Answer 1

There kind of is, but it builds off of molecular orbital theory and is very difficult to articulate in an accessible way.

The core premises of this method are:

• In a molecule with more than two atoms, any orbitals with directional aspects (i.e. not spheres) pointing towards the central atom are considered to be along the `\mathbf(y)` axis (rather than the `z` axis).
• Each atom surrounding a central atom is considered to have its own set of axes. So, each atom has different `x` and `z` axes.
• The orbitals of the surrounding atoms are considered to "transform" as "group orbitals", meaning that they bond to the atoms of the central atom as a group.
• As usual, only compatible orbitals can overlap with each other (just like in MO theory).

As a brief overview of the idea of individual axes, consider this image of a tetrahedral set of axes:

This gets complicated very quickly, so I'll simply work with `"NH"_3`, a relatively simple but not overly simple case (ask if you need help). Recall that this is a trigonal pyramidal molecule.

We will be using a method called Symmetry-Adapted Linear Combinations (SALCs).

What that means is that we'll determine how to categorize certain orbitals to determine which ones are allowed to overlap. Then, we'll build the MO diagram from scratch.

The overall steps we'll be following are:

1. Finding the point group.
2. Finding the reducible representation based on how the hydrogen atoms behave.
3. Reducing the reducible representation to its irreducible representations.
4. Assigning those to the group of hydrogens.
5. Determining which orbitals are compatible.
6. Making the MO diagram.

SYMMETRY CONSIDERATIONS

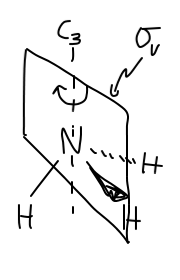
Ammonia belongs to what is called the `C_(3v)` "point group", because it has a principal, `C_3` rotation axis, and because it has a vertical mirror plane `sigma_v`.

A `hatC_3` operation is when you rotate `360^@/3 = 120^@` and achieve the original molecule back. It is the `C_3` symmetry element that acts as the `\mathbf(z)` axis of `"NH"_3`. This is a common convention.

A `hatsigma_v` operation is when you reflect through a mirror plane that is vertically aligned. A `sigma_v` mirror plane is colinear with the `z` axis (if it was perpendicular it would be a `sigma_h`).

DETERMINING THE REDUCIBLE REPRESENTATION

The irreducible representations, or IRREPs, are what we will use to categorize the ways the hydrogens "behave" around the nitrogen.

Given that `"NH"_3` belongs to the `C_(3v)` point group, it is associated with what's called a character table:

To determine the irreducible representations, we first need the reducible representations, `Gamma` (gamma), for the hydrogens as a group. To do that, we follow these steps:

1. For each symmetry element (`E`, the identity, `C_3(z)`, the principal rotation, and `sigma_v`, the vertical mirror plane), operate on the hydrogen atoms as a group and see where each one ends up.
2. The hydrogens that stay where they are and do NOT move contribute `1` to `Gamma`.
   The hydrogens that DO move contribute `0` to `Gamma`.
   The hydrogens that change orbital sign contribute `-1` to `Gamma`.

Now, let's align the molecule in a more convenient way. Let the `z` axis be pointing towards you. Thus, as we said before, the `C_3` axis IS the `z` axis.

We see that there are three `sigma_v` mirror planes (`a,b,c`) and three hydrogens (`H_a`, `H_b`, `H_c`). Now, let's see what happens if we perform each symmetry operation.

`hatE`:

• `color(green)(H_a -> H_a)`
• `color(green)(H_b -> H_b)`
• `color(green)(H_c -> H_c)`

Thus, we get `1 + 1 + 1 = color(blue)(3)` for the first number in `Gamma` for the `E` operation (the identity operation).

`hatC_3(z)` (counterclockwise):

• `H_a -> H_b`
• `H_b -> H_c`
• `H_c -> H_a`

Each hydrogen moved, and none of them stayed where they are, so this operation contributes `color(blue)(0)` to `Gamma`.

`hatsigma_v`:

• `color(green)(H_a -> H_a)`
• `H_b harr H_c`

`H_a` stayed in place, but `H_b` and `H_c` swapped places. So, there is a contribution of `color(blue)(1)` to `Gamma`.

Since ammonia is symmetrical and each hydrogen is identical, performing `hatsigma_v(a)`, `hatsigma_v(b)`, and `hatsigma_v(c)` each do the same thing, so we do not need to do all three.

The reducible representation then, using the `1s` atomic orbitals of hydrogen as a basis, is written as:

`color(blue)(" "" "" "E" "C_3(z)" "sigma_v)`
`color(blue)(Gamma_(1s) = " 3"" "0" "" "" "1)`

Phew, now let's reduce this!

THE IRREDUCIBLE REPRESENTATIONS

Basically, the rows in the character table above have to add up to give `Gamma`. The result becomes:

So, the group orbitals of hydrogen "transform under `A_1` and `E` symmetry". What does THAT mean? It means that they can either symmetrically behave one at a time (`A_1`), or two at a time (`E`).

This is depicted as follows:

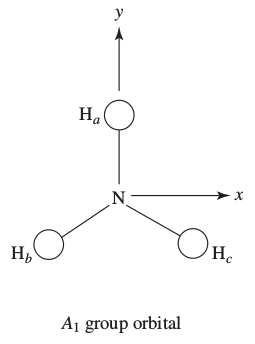
In this one, the hydrogen `1s` orbitals are all the same sign, and no matter what happens, they stay the same sign.

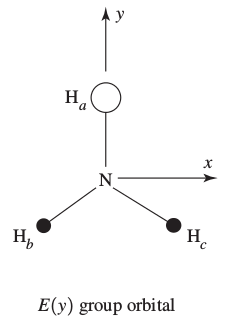
In this one, there is one hydrogen `1s` orbital that is the opposite sign to the others, and a horizontal node exists.

This one is similar to `E(y)`, but the node is perpendicular to the horizontal node in `E(x)` (the third hydrogen doesn't matter here).

FINALLY DRAWING THE MO DIAGRAM!

Okay, finally, we have enough information to draw an approximate MO diagram.

To save time (and brain), I'm just going to have to tell you the symmetries of nitrogen's orbitals. The `2s` is `A_1`, the `2p_z` is `A_1`, and the `2p_y` and `2p_x` are collectively `E`.

The orbitals will combine like so:

• `"3H"` `A_1` with `"N"` `A_1` (bonding, antibonding)
• `"3H"` `E(x)` with `"N"` `E` (bonding, antibonding)
• `"3H"` `E(y)` with `"N"` `E` (bonding, antibonding))
• `"Nothing"` with `"N"` `A_1` (nonbonding)

Using the orbital potential energies (Appendix B.9) of each atom, we eventually get:

Note that we came in with seven orbitals (`3xxH + "N"_(2s) + "N"_(2p_x) + "N"_(2p_y) + "N"_(2pz)`) and came out with seven (`2a_1`, `3a_1`, `4a_1`, `2xx1e`, `2xx2e`).

Also note that only the orbitals of the same symmetry (`A_1` with `A_1`, `E` with `E`, etc.) can interact with each other---in other words, they are compatible. You can trace that by looking at the dashed lines.

The first three bonding MOs of `"NH"_3` account for the three single bonds, and the highest-energy MO holds the lone pair of electrons on nitrogen (it is fairly nonbonding).

And here is a reference (Inorganic Chemistry, Miessler et al., pg. 156) to show the actual MO diagram.