Explain the forces acting, including friction, when an object is stationary or moving on an inclined plane. What coordinate system do we use?
2 Answers
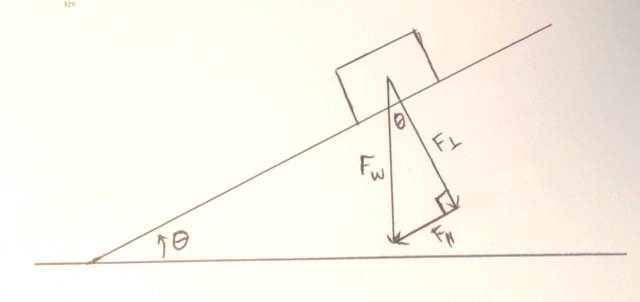
Three forces act:
Explanation:
The forces acting are a normal force at right angles to the plane, an accelerating force parallel to the plane and 'downhill', and a frictional force parallel to the plane and 'uphill'. The fundamental cause of all these forces is the weight force on the object, acting directly downward toward the centre of the earth.
The gravitational force is the key to the whole situation. It is given by
Using the image above, note that the slope is at an angle
The constructed triangle is a right-angled triangle, so we can use trigonometry.
Using the definitions
Finally, the frictional force will act in the opposite direction to
By rotating the axes to be aligned with the plane.
Explanation:
You take the axes and align them with the plane, so that the coordinates are 'parallel to the plane and perpendicular to the plane', rather than 'horizontal and vertical':
 https://www.ilephysique.net/sujet-mouvement-sur-un-plan-incline-246708.html
https://www.ilephysique.net/sujet-mouvement-sur-un-plan-incline-246708.html
You draw the forces involved:
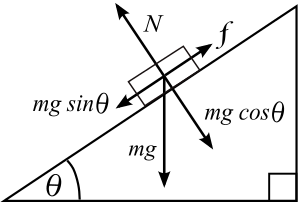 https://fr.wikipedia.org/wiki/Plan_incliné
https://fr.wikipedia.org/wiki/Plan_incliné
If your object is not moving use Newton's first law:
If your object is moving use Newton's second law:

