A cone has a height of #18 cm# and its base has a radius of #7 cm#. If the cone is horizontally cut into two segments #8 cm# from the base, what would the surface area of the bottom segment be?

Question

946 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-04-21T09:44:02

#:.color(purple)(=447.6cm^2# to the nearest 1 decimal place #

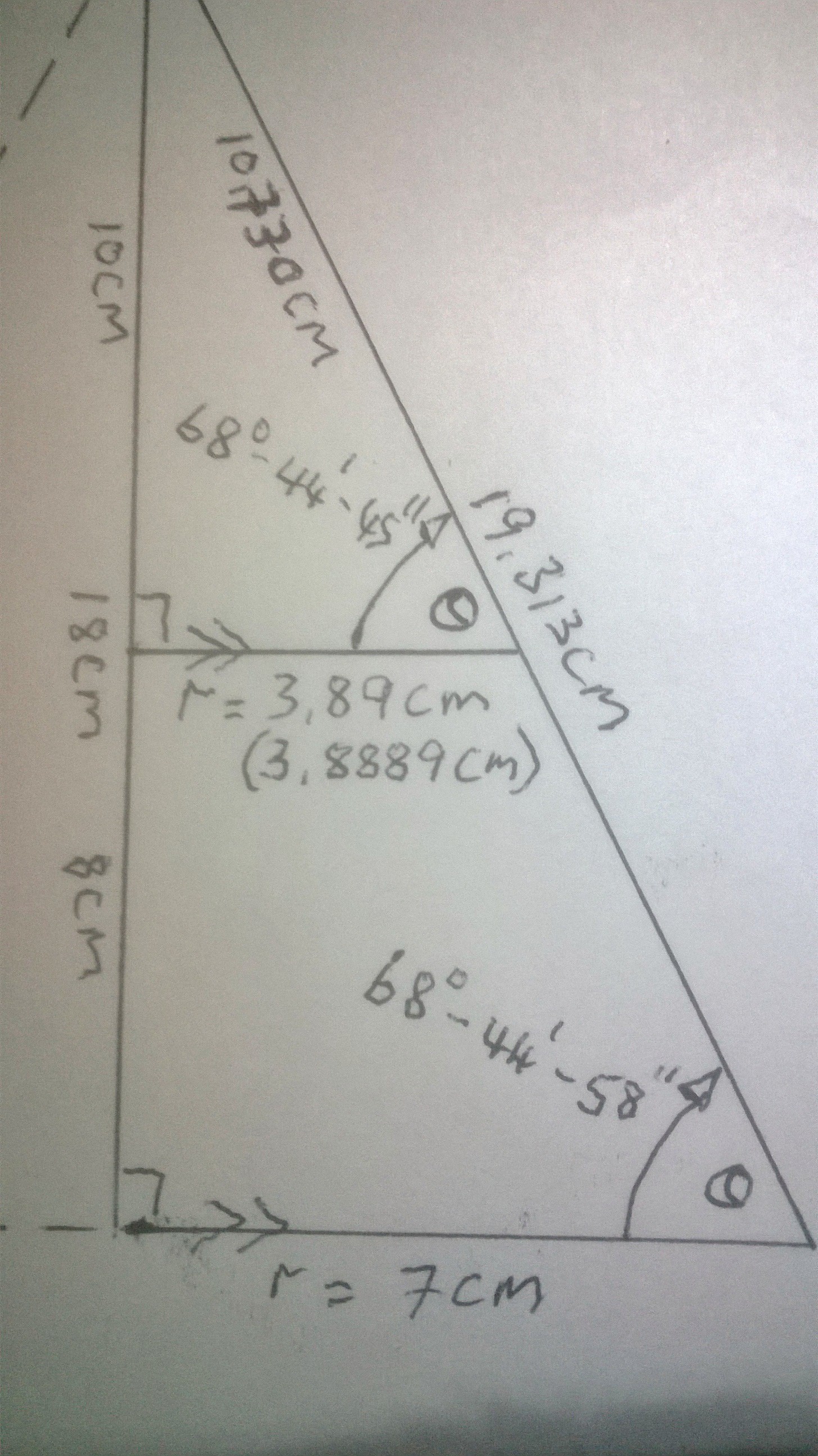
enter image source here

:.Pythagoras: #c^2=18^2+7^2#

#:.c=L=sqrt(18^2+7^2)#

#:. c=Lcolor(purple)(=19.313cm#

#:.18/7=tan theta=2.571428571=68^@44’58”#

:.#color(purple)(S.A.#= pir^2+pir*L#

:.S.A.#=3.141592654*7^2+pi*7*19.313#

:.S.A.#=153.93804+424.715#

:.Total S.A.#color(purple)(=578.653cm^2#

#:.Cot 68^@44’58”*10=3.89cm=#radius of top part

:.Pythagoras: #c^2=10^2+3.89^2#

#:.c=L=sqrt(10^2+3.89^2)#

#:. c=Lcolor(purple)(=10.730cm# top part

:.S.A. top part#=pi*r^2+pi*r*L#

S.A. top part#:.3.141592654*3.8889^2+pi*3.8889*10.730#

S.A. top part#:.=47.512+131.092#

S.A. top part#:.color(purple)(=178.604cm^2#

:.S.A. Botom part#color(purple)(=578.653-178.604=400.049cm^2#

The total surface area of the bottom part got to include
the surface area of the circle of the top part.

:.S.A. Botom part:.=47.512+400.049=447.561 cm^2#

#:.color(purple)(=447.6cm^2# to the nearest 1 decimal place #