A projectile is shot from the ground at an angle of #pi/6 # and a speed of #3 /2 m/s#. Factoring in both horizontal and vertical movement, what will the projectile's distance from the starting point be when it reaches its maximum height?
1 Answer
Jan 14, 2016
Explanation:
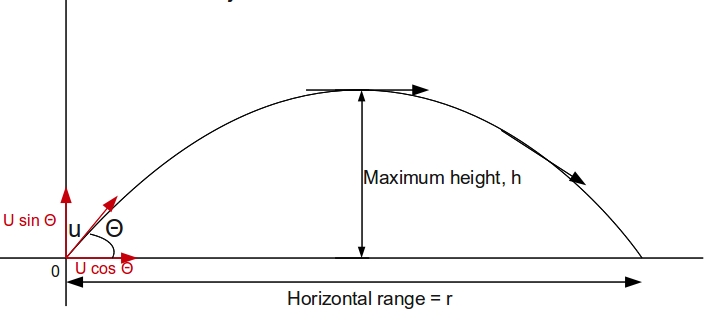
Step 1: Maximum height will be reached when
Use the formula
Given
Take
Since gravity is acting against the velocity so it is deceleration and
Step 2:Distance traveled horizontally in time t is due to
