A triangle has corners at #(-2 ,3 )#, #(3 ,2 )#, and #(5 ,-6 )#. If the triangle is dilated by a factor of #2 # about point #(1 ,-8 ), how far will its centroid move?

1 Answer
ali ergin
Dec 6, 2016

#d=7.73" (shown in figure)"#

Explanation:

#"The original triangle and its centroid is shown in figure below."#

enter image source here

#"the original centroid can be calculated using:"#

#x=(-2+3+5)/3=6/3=2#

#y=(3+2+-6)/3=-1/3=-0,33#

#E(2,-0.33)#

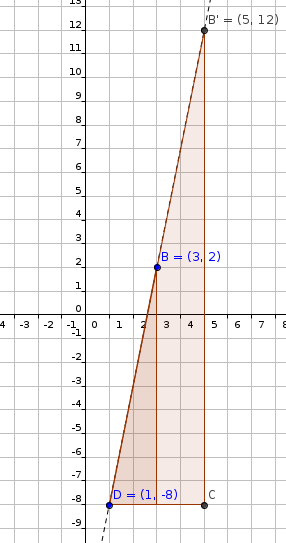
#"Now dilate A(-2,3) by factor 2 with respect to D(1,-8)"#

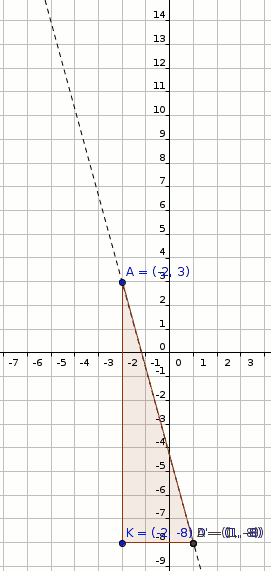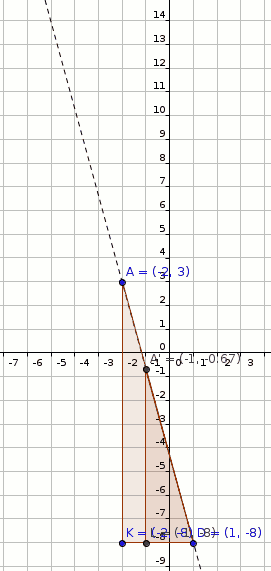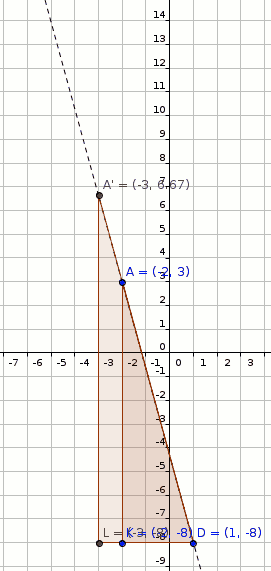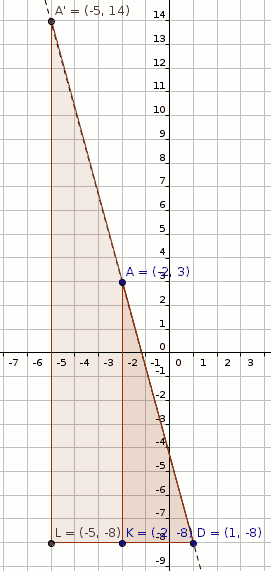
enter image source here

#A(-2,3) rArr A'(1-3*2,3+11*2)#

#A'(1-3*2,-8+11*2)#

#A'(-5,14)" (shown in figure below)"#

#![enter image source here](https://useruploads.socratic.org/v1gFJeqkTVal4HBb8JJA_P2.png) #

#"Dilate B(3,2) by factor 2 with respect to D(1,-8)"#

enter image source here

#B(3,2) rArr B'(1+2*2,-8+10*2)#

#B'(1+2*2,-8+10*2)#

#B'(5,12)" (shown in figure below)"#

enter image source here

#"Dilate C(5,-6) by factor 2 with respect to D(1,-8)"#

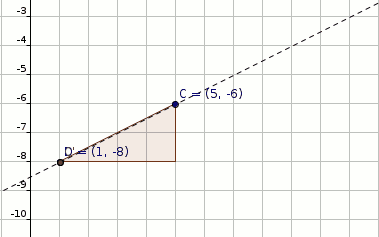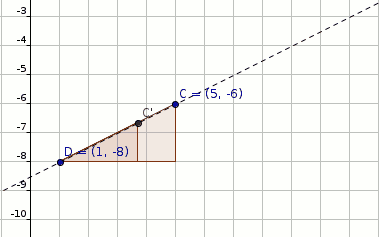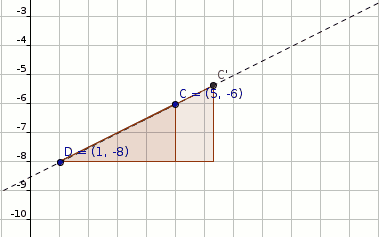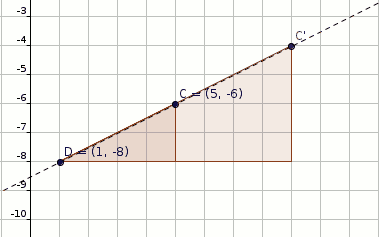
enter image source here

#C(5,-6) rArr C'(1+4*2,-8+2*2)#

#C'(1+4*2,-8+2*2)#

#C'(9,-4)" (shown figure below)"#

enter image source here

#"Finally.."#

#"the dilated centroid can be calculated " #

#x'=(-5+5+9)/3=9/3=3#

#y'=(14+12-4)/3#

#y'=22/3=7,33#

#F(3,7.33)#

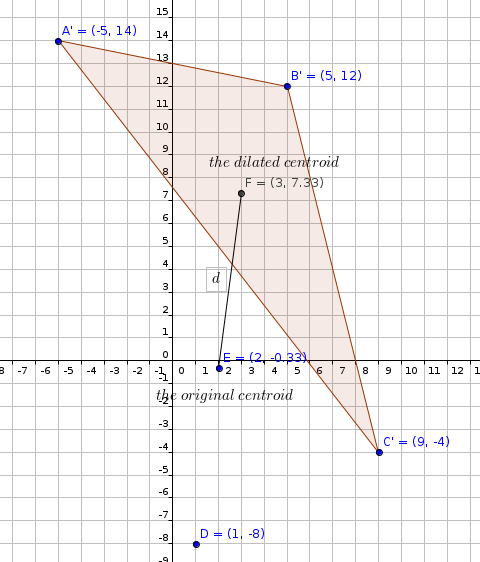
enter image source here

#"distance between E and F"#

#d=sqrt((3-2)^2+(7.33+0.33)^2)#

#d=sqrt(1+(7.66)^2)#

#d=sqrt(1+58.68)#

#d=sqrt(59.68)#

#d=7.73#

Related questions
See all questions in Dilations or Scaling around a Point
Impact of this question
1040 views around the world
You can reuse this answer
Creative Commons License