A triangle has corners at #(6 ,4 )#, #(7 ,6 )#, and #(3 ,3 )#. What is the area of the triangle's circumscribed circle?
2 Answers
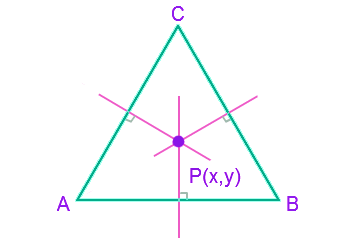
Let the radius of the circumscribed circle be r.
The formula for calculating the radius r is
Where
Where,
Plug in the values and get the answer.
Once you have the radius, you can easily find out the area of the circle.
Area of the circumcircle = 39.2692
Explanation:
Circumcenter of a triangle is a point where all the three perpendicular bisectors of the sides concur. Distance between the vertices and the Circumcenter is the radius of the circumcircle.
Steps to find the area of the circumcircle
1. Find and calculate the mid point of the sides AB, BC, CA.
2. Calculate the slope of the particular line.
3. By using the midpoint and the slope, find the equation of the perpendicular bisector.
4. Find the equation of other perpendicular bisectors.
5. Solve 2 bisector equations to find out the intersection point (circumcenter.
6. Find the distance between circumcenter and one vertex of the triangle to find the radius of the circumcircle.
7. Having known the radius, area of the circumcircle is given by formula
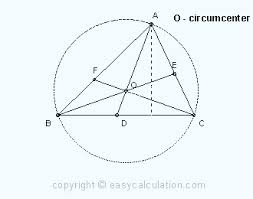
D coordinates
Slope of line BC
Slope of perpendicular bisector through point D
Equation of perpendicular bisector through point D
E coordinates
Slope of line AC
Slope of perpendicular bisector through point E
Equation of perpendicular bisector through point E
Solve Eqns (1) & (2) to get circumcenter (O) coordinates.
Circumcircle radius is OA = OB = OC
Verification of radius
Area of circumcircle =