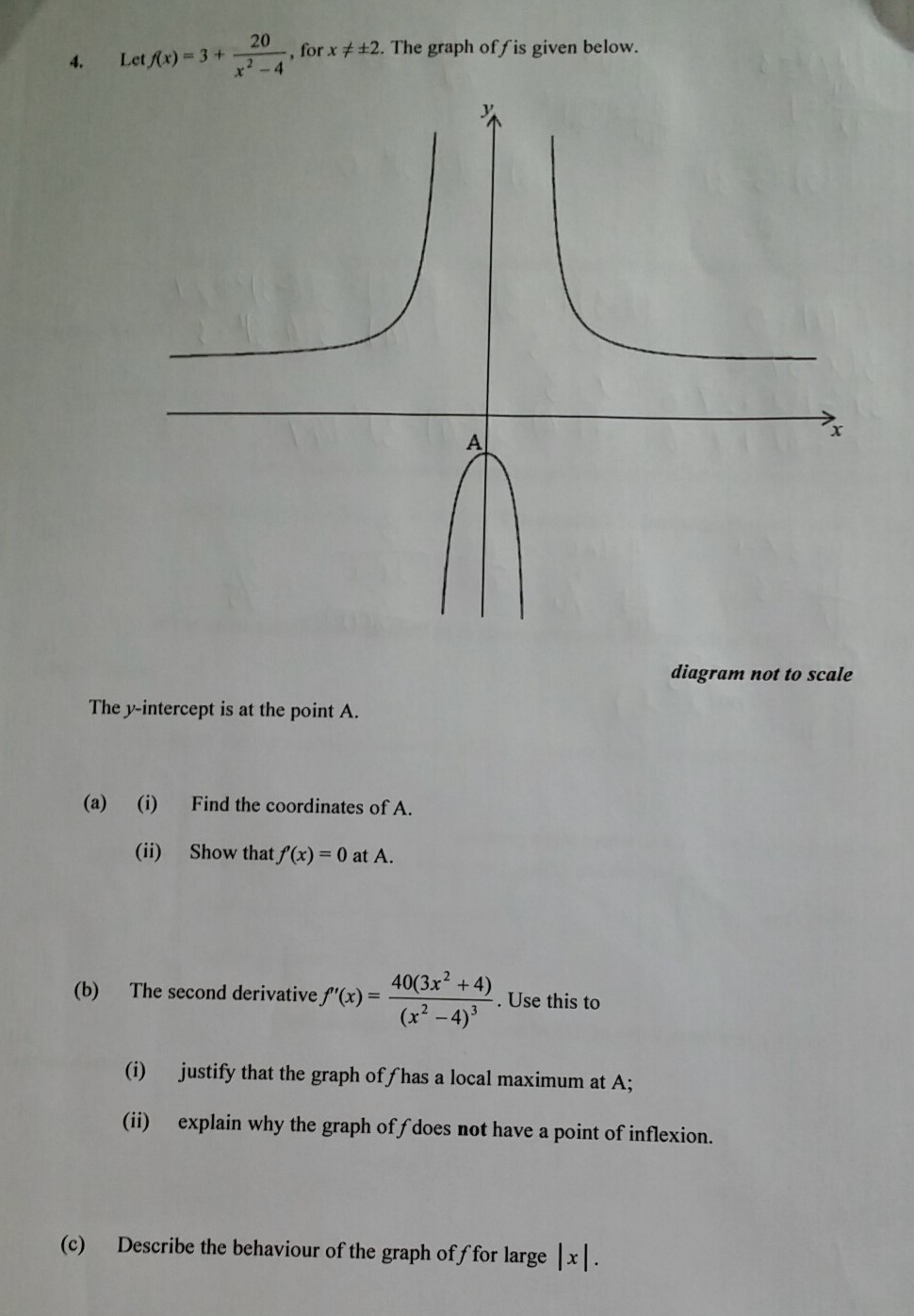
#f(x) = 3+20/(x^2-4)#
(a)
(i)
Clearly the point #A# is on the y-axis, so #x=0.#
For the y-coordinate find #f(0)#:
#f(0)=3+20/(0^2-4)=3-5=-2#
So #A# is at #(0,-2)#.
(ii)
#f(x) = 3+20(x^2-4)^(-1)#
#->f'(x) =-2x*20(x^2-4)^-2#
#= 2x*(-20)/(x^2-4)^2=(-40x)/(x^2-4)^2#
#f'(0) = (-40(0))/((0)^2-4)^2=0#
(b)
(i)
#f''(x)=(40(3x^2+4))/(x^2-4)^3#
#f''(0)=(40(3(0)^2+4))/((0)^2-4)^3=160/-64=-5/2<0#
Since #f''(0)<0# then the point must be a maximum.
(ii)
Set #f''(x)=0# then:
#(40(3x^2+4))/(x^2-4)^3=0#
#->3x^2+4=0#
which clearly has no real roots. In order for a point of inflection to exist #f''(x)=0# for some real value of #x#. But we has just shown that cannot happen.
(c)
From the graph we can clearly see horizontal asymptotes at #y=3# for very large #|x|#. If we look at the function:
#f(x) = 3+20/(x^2-4)#
The #x^2# term on the denominator of the fraction makes the fractional term very small for very large |x| so we can say that:
#f(x)=3+20/(x^2-4)->3# as #x# gets very large. Hence it behaves like a constant at #3# as demonstrated on the plot.