Charges of +2microC, +3microC and -8microC are placed in air at the vertices of an equilateral triangle of ide 10cm.What is the magnitude of the force acting on the -8microC due to the other two charges?
1 Answer
Mar 14, 2018
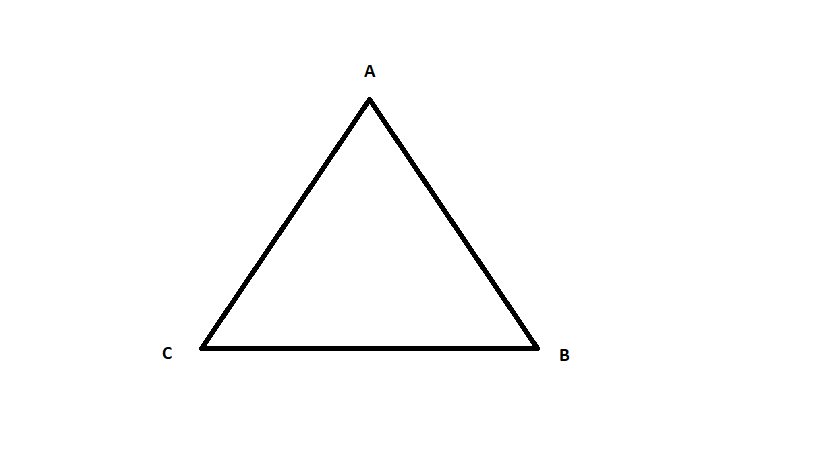
Let charge
So,net force on
and the value is
And due to
So,two forces of
Making an angle of

