Question

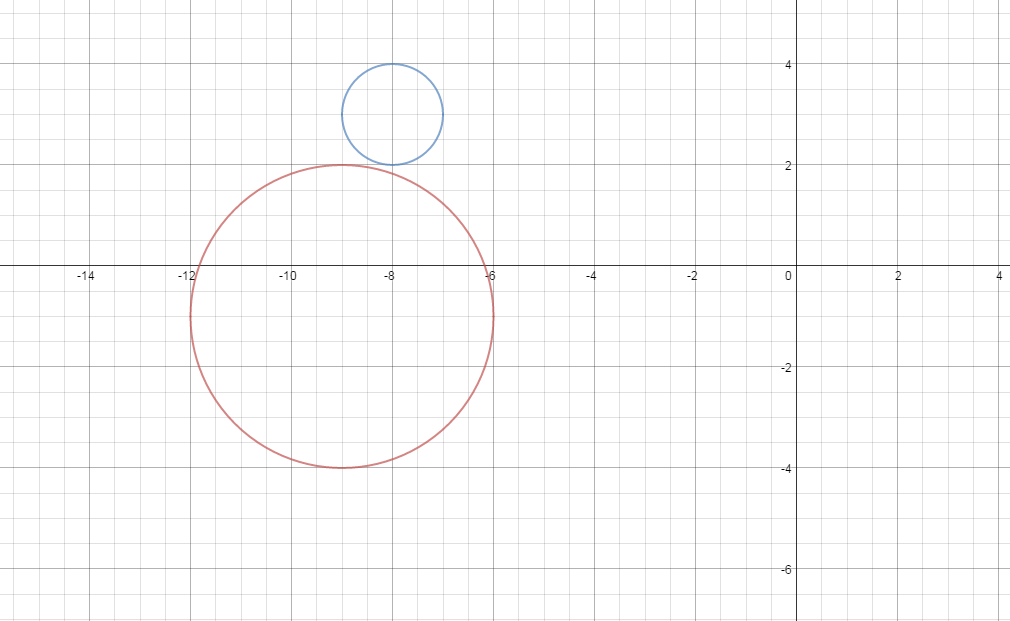
Circle A has a center at `(-9 ,-1 )` and a radius of `3`. Circle B has a center at `(-8 ,3 )` and a radius of `1`. Do the circles overlap? If not what is the smallest distance between them?

Answer 1

The circles do not overlap.
Smallest distance between them`=sqrt17-4=0.1231`

Explanation:

From the given data:
Circle A has a center at (−9,−1) and a radius of 3 . Circle B has a center at (−8,3) and a radius of 1
. Do the circles overlap? If not what is the smallest distance between them?

Solution: Compute the distance from center of circle A to center of circle B.

`d=sqrt((x_a-x_b)^2+(y_a-y_b)^2)`

`d=sqrt((-9--8)^2+(-1-3)^2)`

`d=sqrt((-1)^2+(-4)^2)`

`d=sqrt(1+16)`

`d=sqrt17`

`d=4.1231`

Compute the sum of the radii :

`S=r_a+r_b=3+1=4`

Smallest distance between them`=sqrt17-4=0.1231`

God bless....I hope the explanation is useful.