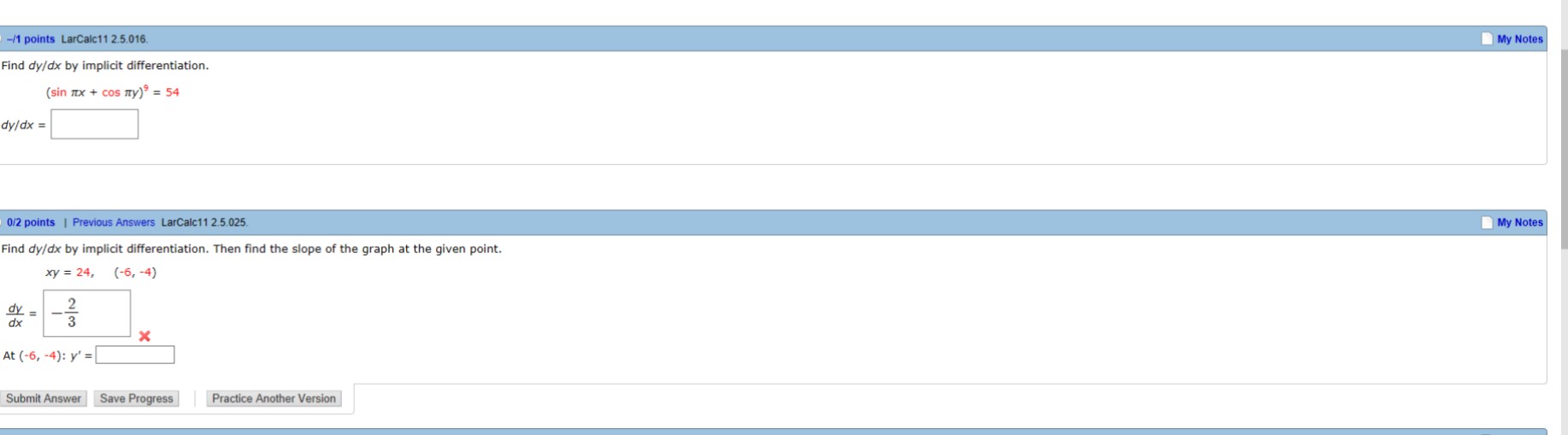
Find dy/dx by implicit differentiation. Then find the slope of the graph at the given point. ? xy = 24, (-6, -4)

Question

4047 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-02-08T06:22:25

The slope is #=-2/3#

The function is

#xy=24#

#(uv)'=u'v+uv'#

#u=x#, #=>#, #u'=1#

#v=y#, #=>#, #v'=dy/dx#

#(24)'=0#

Therefore,

#(xy)'=(24)'#

#1*y+x*dy/dx=0#

#dy/dx=-y/x#

At the point #(x,y)=(-6,-4)#

#dy/dx=-(-4)/(-6)=-4/6=-2/3#

graph{(xy-24)(y+4+2/3(x+6))=0 [-26.61, 13.96, -12.3, 7.97]}