Find the arc length for the following question?

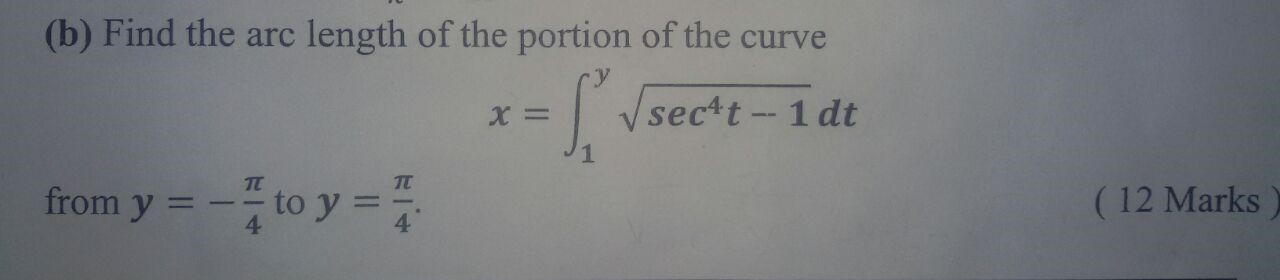
enter image source here

1 Answer
Ultrilliam
May 7, 2018

#2#

Explanation:

For #x = x(y)#, arc length is #s = int_(y_1)^(y_2) \ sqrt(1 + (x')^2) \ dy#

From Fundamental Theorem of Calculus:

  • #x(y) = int_1^y \ sqrt (sec^4 t - 1 ) \ dt implies x' = sqrt (sec^4 y - 1 )#

So:

#s = int_(- pi/4 )^(pi/4) \ sqrt(1 + (sqrt (sec^4 y - 1 ))^2) \ dy#

# = int_(- pi/4 )^(pi/4) \ sec^2 y \ dy#

# = int_(- pi/4 )^(pi/4) \ (tan y)^' \ dy#

# = ( \ tan y )_(- pi/4 )^(pi/4)= 2#

Related questions
Impact of this question
1127 views around the world
You can reuse this answer
Creative Commons License