Question

Find value(s) c ∈ (−2, 4) such that f'(c) is parallel to the chord line joining ?

• 

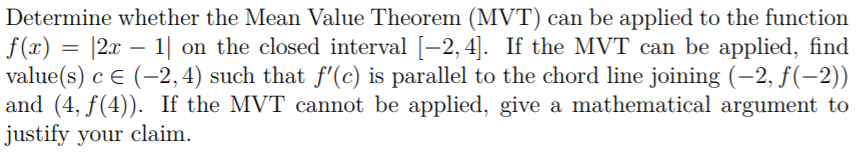
Im not sure wich Theorem they mean :/

Answer 1

Mean Value Theorem
If a function `f` is continuous on `[a,b]` and differentiable on `(a,b)`,
then there exists c in `(a,b)` such that `f'(c)={f(b)-f(a)}/{b-a}`.

Explanation:

You can learn more about the theorem here: https://socratic.org/calculus/graphing-with-the-first-derivative/mean-value-theorem-for-continuous-functions

In this particular question, `f` is continuous on `[-2,4]`, but not differentiable on `(-2,4)` because the derivative does not exist at `1/2`.

In this case there is no point in the interval at which the tangent line is parallel to the secant line (chord).