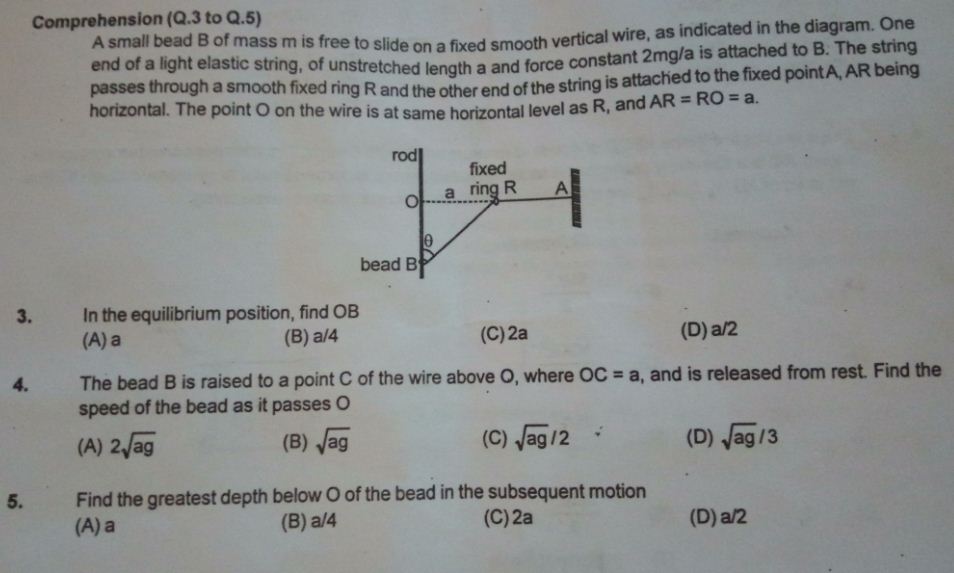
3. At the point #B#, the length of the elastic string is #a+a csc theta#, and hence the elongation is #a csc theta#. So, the force exerted by the string on the bead is #(2mg)/a a csc theta = 2mg csc theta#
For the net vertical component of the force on the bead to be zero, we have
#2mg csc theta times cos theta = mg implies #cot theta =1/2#
So, #OB= a cot theta =a/2#
4. At the point #C#, the elongation of the spring is #a sqrt 2#, so that the elastic potential energy at that point is
#1/2 times (2mg)/a times (a sqrt2)^2 = 2mga#
The gravitational potential energy of the bead at that point is #mga# (assuming the reference level of this energy to be at #O#).
At the point #a#, the elastic potential energy is
#1/2 times (2mg)/a times a^2 = mga#
So, energy conservation gives
#2mga+mga = mga+1/2mv^2 implies v = 2 sqrt(ag)#
5. Let the greatest depth that the bead reaches be #d#. At the lowest point, the elongation of the string is #sqrt(a^2+d^2)# and so the net potential energy is
#1/2 times (2mg)/a times (a^2+d^2)-mgd#
At this point, the bead has to be at rest, so conservation of energy yields
#3mga = (mg)/a(a^2+d^2)-mgd implies d^2-ad-2a^2=0implies#
#(d+a)(d-2a) = 0 implies d = 2a#