Question

Half of the chain is lying on the table. The rest of the chain hangs in equilibrium from both of the ends of the table. The extra length of the hanging chain at one end is `1/8`th of the total length of the chain than that hanging at the other end?

Find the minimum value of the coefficient of friction between table and chain.

Answer 1

0.5

Explanation:

Let's assume,mass of the total chain is `m`,so the part lying on the table will have a mass of `m/2`,the two parts hanging from the edge of the table will be`m/8` and `3m/8` respectively(refer to the diagram)

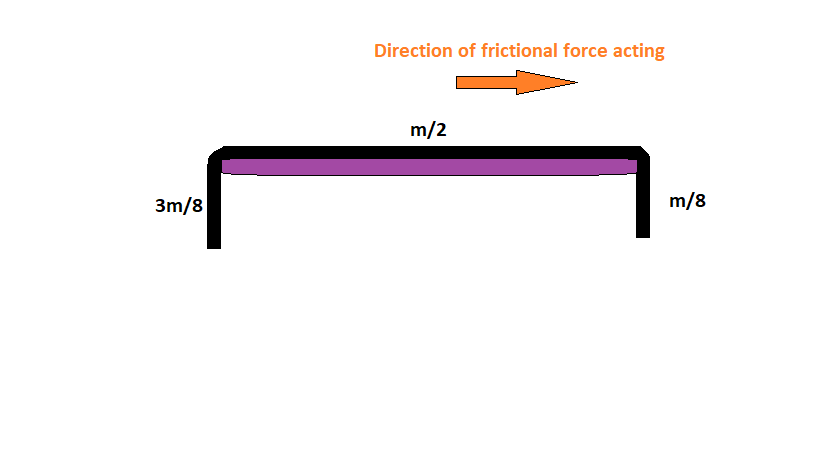
so,the pulling force of the part of chain`(F1)` having mass `3m/8` must be balanced by the frictional force`(f)` acting on the part lying on the table and that one hanging from the other end to be in equilibrium.`(F2)`

now,frictional force will act in the shown direction as the net tendency of the system is to move along the mass of chain hanging from the edge of the table with mass `3m/8`

so,we can write,
`F1=F2+f`
or, `3/8mg` = `m/8g` + `mu` `(m/2)g` (where `mu` is the desired coefficient of friction)

solving we get, `mu`=0.5