Help with waterwaves?
In a vessel with water stands a vertical wall AB that extends over the water surface. On one side of AB is a rectangular plate ABCD in the bottom of the vessel. The water is therefore more shallow in the area of ABCD than on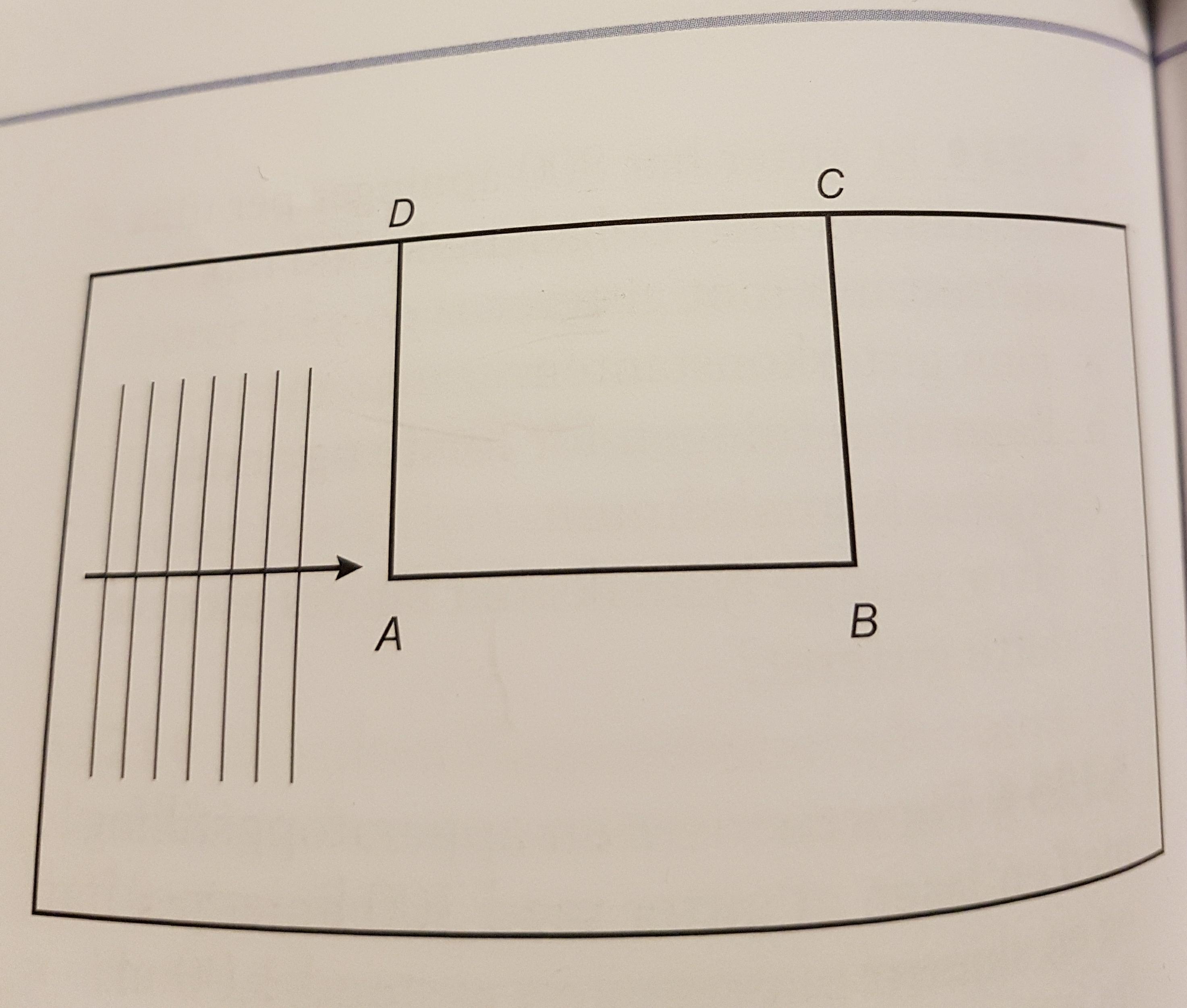
the vessel. From the left part of the vessel, straight-line water waves are sent at a speed direction parallel to AB. In the deep water the speed is equal to 0.3m / s and the wavelength is equal to 6.0cm, while the speed is equal to 0.2m / s in the shallow water. We assume that the waves are not reflected from the walls of the vessel.
Find out if the waves that have gone on either side of AB are in phase in the right part of the vessel when 1) AB = 0.6m 2) AB = 0.80m
In a vessel with water stands a vertical wall AB that extends over the water surface. On one side of AB is a rectangular plate ABCD in the bottom of the vessel. The water is therefore more shallow in the area of ABCD than on the vessel. From the left part of the vessel, straight-line water waves are sent at a speed direction parallel to AB. In the deep water the speed is equal to 0.3m / s and the wavelength is equal to 6.0cm, while the speed is equal to 0.2m / s in the shallow water. We assume that the waves are not reflected from the walls of the vessel.
Find out if the waves that have gone on either side of AB are in phase in the right part of the vessel when 1) AB = 0.6m 2) AB = 0.80m
1 Answer
A In phase
B Out of phase
Explanation:
The formula that relates wave velocity, v; wavelength,
Therefore we can find the frequency of the wave using the data given of v and
The frequency will also be 5 Hz in the slow water, so the wavelength and velocity are directly proportional. Therefore the wavelength in the shallow water area is given by
Case A: AB = 0.6 m -- As the waves travel from A to B, how many cycles of the waves are there?
In the deep water, there are 0.6 m/0.06 m = 10 complete cycles.
In the shallow water, there are 0.6/0.04 m = 15 complete cycles.
The important thing to this question is that on both sides of AB, the last cycle on both sides is at the same part of the wavelength when they rejoin. In this case, the last cycle on both sides completed right at the end of wall AB.
The peaks and troughs will be side by side when the waves that have traveled over the plate ABCD rejoin those that were outside the ABCD area -- in phase.
Case B: AB = 0.4 m -- As the waves travel from A to B, how many cycles of the waves are there?
In the deep water, there are 0.4 m/0.06 m = 6 complete cycles and 2/3 of a 7th cycle.
In the shallow water, there are 0.4/0.04 m = 10 complete cycles.
The peaks and troughs will not be side by side when the waves that have traveled over the plate ABCD rejoin those that were outside the ABCD area -- out of phase.
To explain case B a bit more completely, the waves that traveled in more shallow water complete the last cycle (the 10th cycle) at the end of the wall. On the deeper side, the last cycle was only 2/3 complete at the end of the wall.
I hope this helps,
Steve
