Question

How are hyperbolic sine, hyperbolic cosine, and hyperbolic tangent used in real life?

I know everything about them, but I am curious about problems that they could solve in real world.

Answer 1

Catenary Curve...

Explanation:

This is an idea i have heard about a while ago...

Lets say we take a piece of slack string, and hold it, so it is still slack, and so it hangs vertically downward, or even a peice of chain, tethered to two posts, and hangs downward, both of these can be directly modelled by the hyperbolic cosine, `coshx`

http://mathforum.org/mathimages/index.php/Catenary

Or in general the function is: `y = acosh(x/a)`

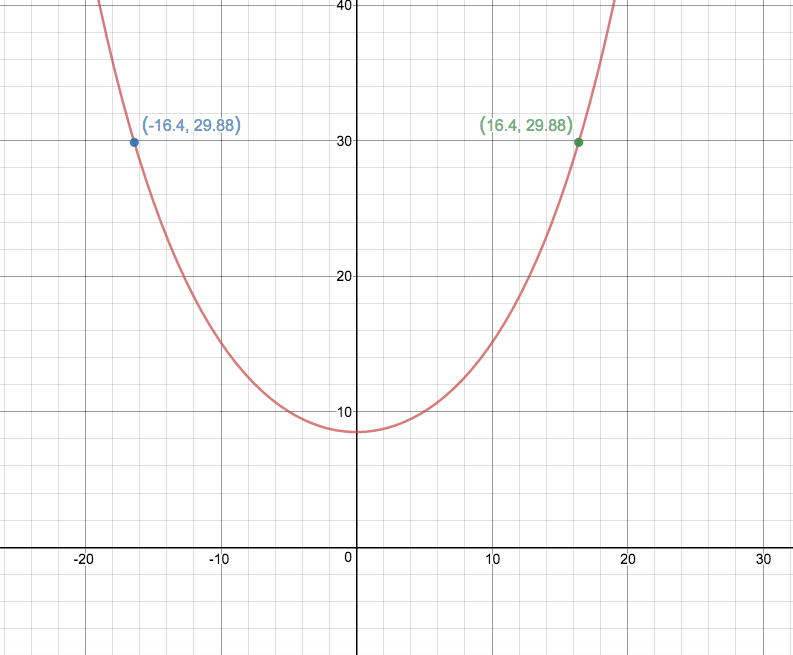
So in this image, it models a peice of string bieng held at `(-16.4,29.88) and (16.4 , 29.88 )`

This is the curve: `y = 8.5cosh(x/8.5)`

Where this curve can be parametrically defined as:

`x(t) = t`
`y(t) = acosh(t/a)`

Where also arcs and archways can also be modelled by the caternary curve, where this has the properties of having a very strong foundation and infrastucture...

http://paulscottinfo.ipage.com/maths-gallery/1/17.st-louis-arch.html

I hope this is a small insight to how hyperbolic functions can be used in the real world!