To construct perpendicular bisectors of a triangle #Delta ABC# you have to consider each side separately as a segment (#AB#, #BC# and #AC#) and construct a perpendicular bisector to each of them.
The easy way to construct a perpendicular bisector #PQ# to segment #AB# is pictured below.
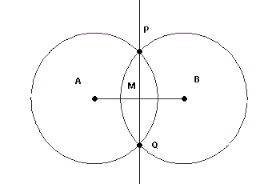
Here the centers of these circles are the endpoints of a given segment #AB# and their radiuses must be the same. The only condition is for these circles is the existence two points of intersection, #P# and #Q#. For this the radius can be any, as long as it's greater than half of the length of #AB#. The simple method is to choose it to be equal to the length of #AB#.
What's more interesting is to prove that this construction delivers the perpendicular bisector.
Here is the proof.
Assume that #M# is an intersection of #AB# and #PQ#.
#AP=BP=AQ=BQ# - each is a radius, which we have chosen
#Delta APQ = Delta BPQ# - by side-side-side theorem
Hence:
#=>/_APQ=/_BPQ# as angles of congruent triangles lying across congruent sides #AQ# and #BQ#
#=> Delta APM = Delta BPM# by side-angle-side theorem
#=> AM=BM# as sides of congruent triangles lying across congruent angles #/_APM=/_BPM#
#=>/_AMP=/_BMP# as angles of congruent triangles lying across congruent sides #AP# and #BP#
#=>/_AMP=/_BMP=90^o# since their sum is #180^o#
So, we have proven that #M# is a modpoint of #AB# and #PM_|_AB#.


