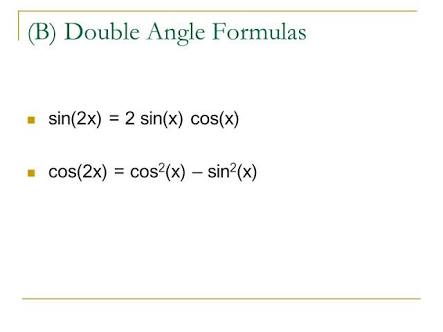
How do you find a double angle formula for sec(2x) in terms of only csc(x) and sec(x)?

Question

78535 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-03-07T13:37:30

See Below.

#sec(2x)#

= #1/cos (2x)#

= #1/(cos (x + x))#

= #1/(cos x * cos x + sin x * sin x)# [Expanded using addition identity]

= #1/((1/sec x) * (1/secx) + (1/csc x) * (1/csc x))#

= #1/((csc^2x + sec^2x)/(sec^2x * sin^2 x))# [Simple Addition]

= #(sec^2 x csc^2x)/(csc^2x + sec^2 x)#

Hope this helps.

Answer 2 · 2018-03-07T14:54:11

#color(blue)(=>(sec^2x * csc^2x) / (csc^2x - sec^2x)#

#sec 2x = 1 / (cos 2x) #

enter image source here

#=> 1 / (cos^2 x - sin^2x)# using double angle formula

# => 1 / (1/sec^2x - 1/csc^2x)#

#=> 1 /( (csc^2x - sec^2x) / (sec^2x csc@^2x))#

# =>(sec^2x * csc^2x) / (csc^2x - sec^2x)#