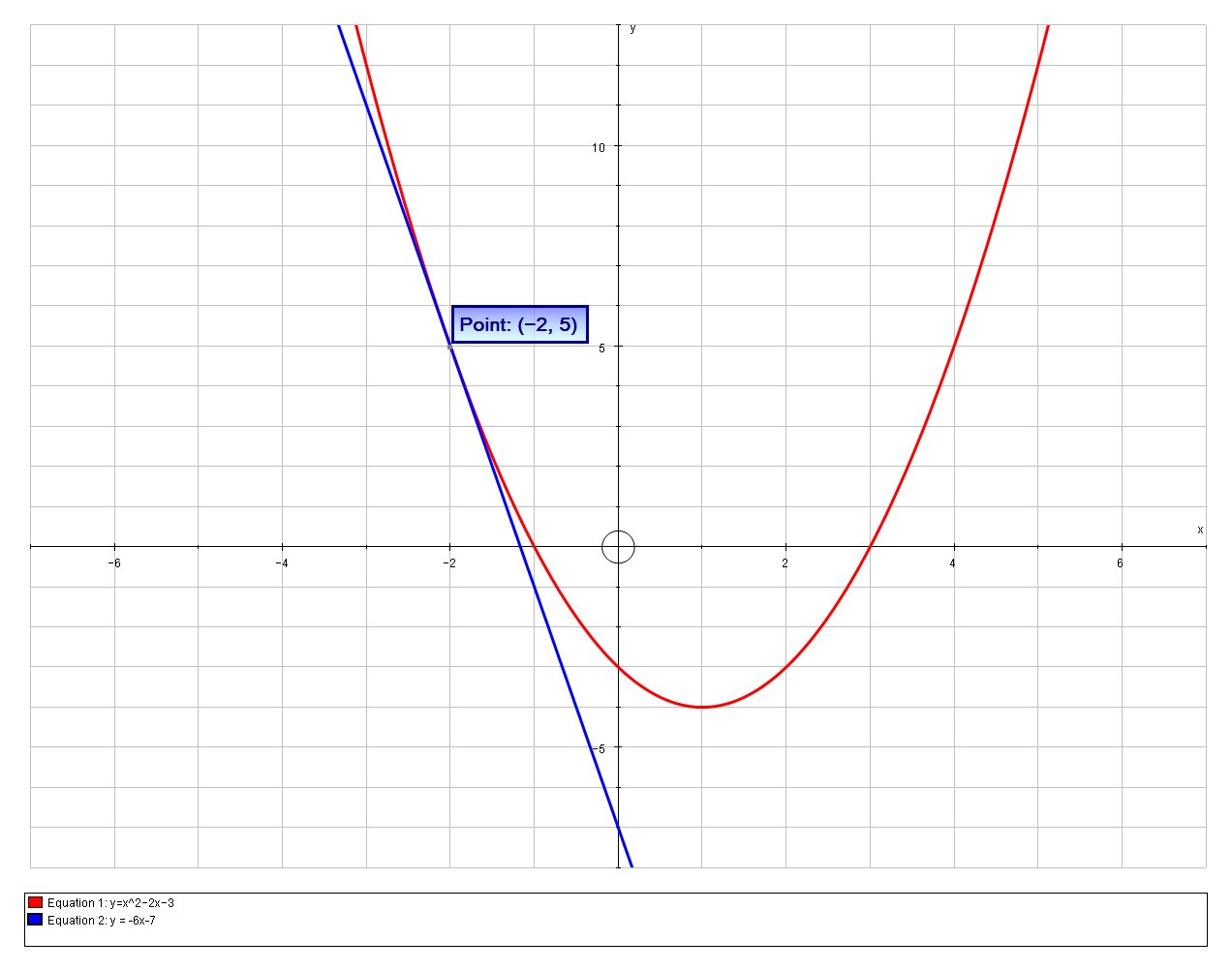
How do you find the equation of the line tangent to #f(x) =x^2-2x-3# at the point (-2,5)?

Question

3828 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-02-25T15:14:31

# y = -6x-7 #

The gradient of the tangent to a curve at any particular point is given by the derivative of the curve at that point.

We have:

# f(x) = x^2-2x-3 #

Differentiating wrt #x# gives:

# f#(x)=2x-2 #

When # \ \ \ \ \ \ \ \ \ \ x=-2 => f'(-2) = -4-2 \ \ = -6#
Check: when #x=2 \ \ \ \ \ =>f(2) \ \ \ \ \ \ =4+4-3=5#

So the tangent passes through #(-2,5)# and has gradient #-6#

Using the point/slope form #y-y_1=m(x-x_1)# the equation of the tangent is:

# y-5 \ \ \ \ \ = -6(x+2) #
# :. y-5 = -6x-12 #
# :. y \ \ \ \ \ \ \ = -6x-7 #

We can verify this graphically:
enter image source here