Question

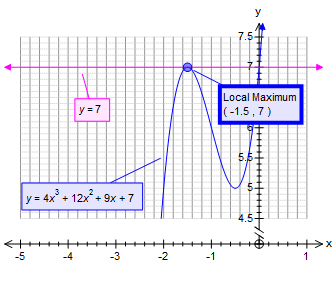
How do you find the equation of the line tangent to `y=4x^3+12x^2+9x+7` at (-3/2,7)?

Answer 1

`y=7`

Explanation:

The slope (gradient) of the line is the rate of change in y for the rate of change in x

So we have `" "("change in y")/("change in x") =(dy)/(dx)`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Let the gradient of the line be `m`
Let any point on the line be P

Given: `" "y=4x^3+12x^2+9x+7`

From this:`" "m=(dy)/(dx) = 12x^2+ 24x+9`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

At the point `(x,y)->(-3/2,7)`

`m=(dy)/(dx)=12(-3/2)^2+24(-3/2)+9`

`m= +27-36+9 = 0`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
So the equation of the tangent is`" " y=(0)x+c`

This tangential line passes through the point `P_(-3/2,7)`

So we have:

`7=(0)(-3/2)+c`

`c=7`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
So the equation of the tangent at `P_(-3/2,7)`

is: `" "y=+7`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~