How do you find the local max and min for #f(x)= sinx#?
1 Answer
Local min/max are at the points where the first derivative of the function are equal to zero.
Explanation:
First Derivative Test
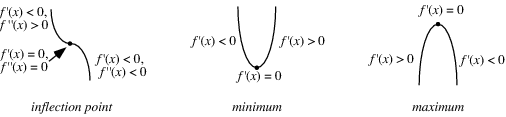
bolded text
Suppose f(x) is continuous at a stationary point x_0.
-
If f^'(x)>0 on an open interval extending left from x_0 and f^'(x)<0 on an open interval extending right from x_0, then f(x) has a local maximum (possibly a global maximum) at x_0.
-
If f^'(x)<0 on an open interval extending left from x_0 and f^'(x)>0 on an open interval extending right from x_0, then f(x) has a local minimum (possibly a global minimum) at x_0.
-
If f^'(x) has the same sign on an open interval extending left from x_0 and on an open interval extending right from x_0, then f(x) has an inflection point at x_0.
Weisstein, Eric W. "First Derivative Test." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/FirstDerivativeTest.html
Second Derivative Test
Suppose f(x) is a function of x that is twice differentiable at a stationary point x_0.
-
If f^('')(x_0)>0, then f has a local minimum at x_0.
-
If f^('')(x_0)<0, then f has a local maximum at x_0.
The extremum test gives slightly more general conditions under which a function with f^('')(x_0)=0 is a maximum or minimum.
If f(x,y) is a two-dimensional function that has a local extremum at a point (x_0,y_0) and has continuous partial derivatives at this point, then f_x(x_0,y_0)=0 and f_y(x_0,y_0)=0. The second partial derivatives test classifies the point as a local maximum or local minimum.
Define the second derivative test discriminant as
D = f_(xx)f_(yy)-f_(xy)f_(yx)
= f_(xx)f_(yy)-f_(xy)^2.
Then
-
If D>0 and f_(xx)(x_0,y_0)>0, the point is a local minimum.
-
If D>0 and f_(xx)(x_0,y_0)<0, the point is a local maximum.
-
If D<0, the point is a saddle point.
-
If D=0, higher order tests must be used.
Weisstein, Eric W. "Second Derivative Test." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/SecondDerivativeTest.html
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 14, 1972.
Thomas, G. B. Jr. and Finney, R. L. "Maxima, Minima, and Saddle Points." §12.8 in Calculus and Analytic Geometry, 8th ed. Reading, MA: Addison-Wesley, pp. 881-891, 1992.

