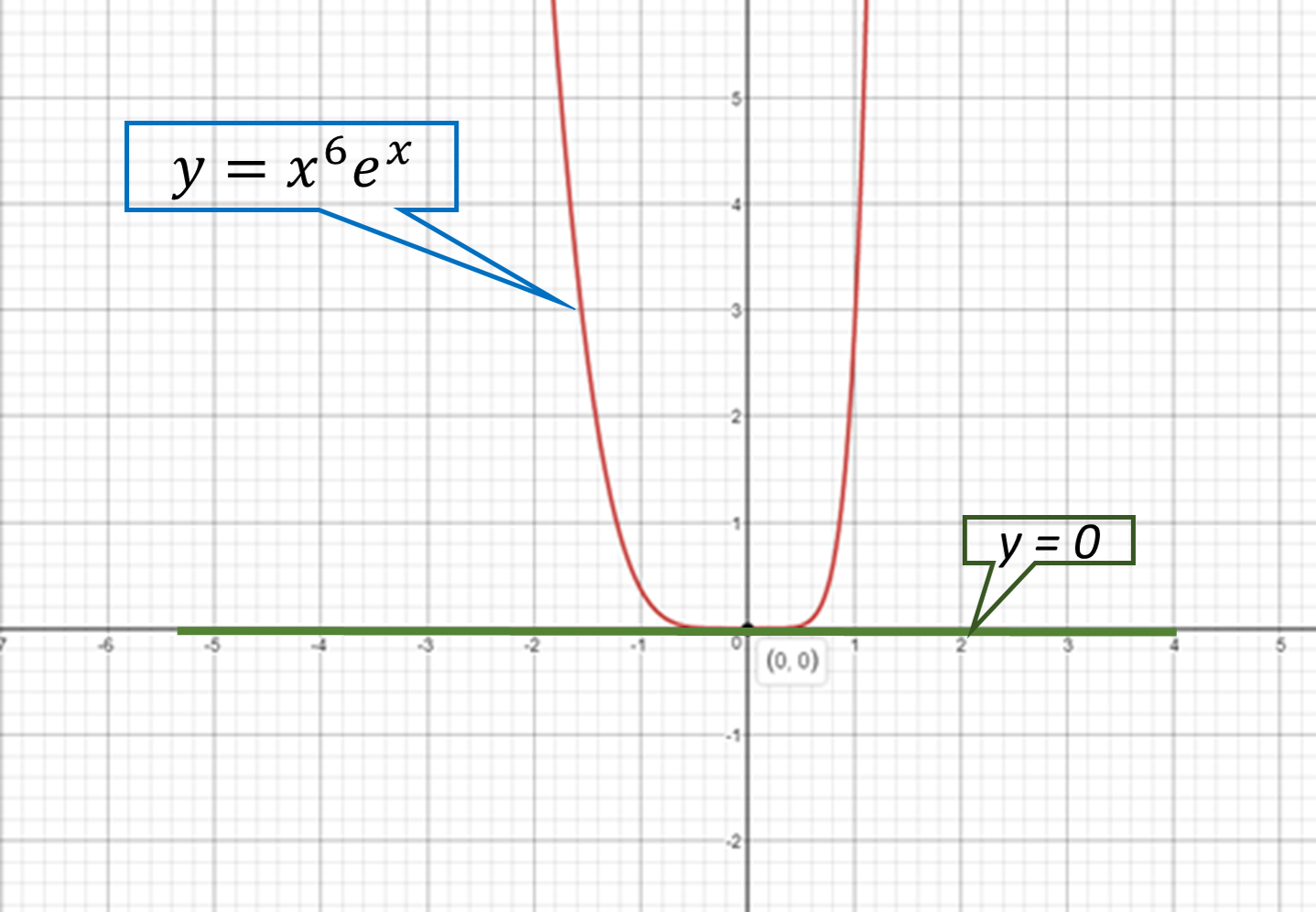
How do you find the points where the graph of the function #y=x^6 * e^x# has horizontal tangents and what is the equation?

Question

4849 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-10-03T05:24:47

The equation of the tangent is #y=0#

Given -

#y=x^6e^x#

Find the slope.

#dy/dx=x^6e^x+e^x.6x^5=x^6e^x+6x^5e^x #

The tangent must draw to a point on the curve where its slope is zero. We have to find the coordinates of that point.

Set first derivative equal to zero.

#x^6e^x+6x^5e^x=0#

Solve it for #x#

#x(x^5e^x+6x^4e^x)=0#
#x=0/(x^5e^x+6x^4e^x)=0#

At #x=0# the slope of the curve is also equal to zero.

The equation of the tangent is #y=0#

enter image source here