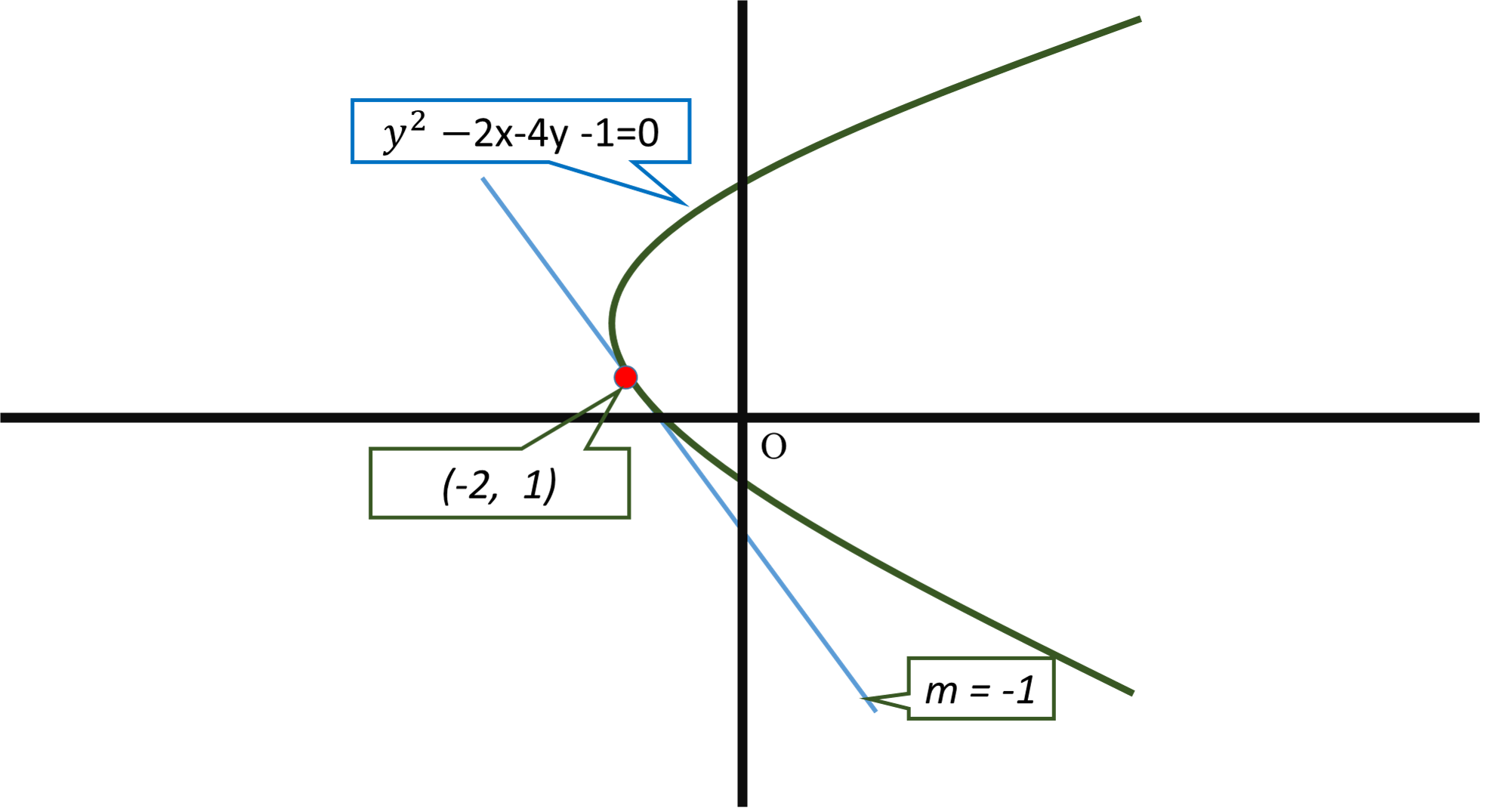
How do you find the slope of a tangent line to the graph of the function #y^2-2x-4y-1=0#, at (-2,1)?

Question

4309 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-10-01T11:39:24

Slope of the tangent is #m=-1#

The first derivative of the function gives the slope of the line at any given point.

Given -

#y^2-2x-4y-1=0#
Differentiate implicitly

#2y dy/dx-2-4dy/dx=0#

#dy/dx(2y-4)=2#

#dy/dx=2/(2y-4)#

Slope at #(-2,1)#

#m=2/(2(1)-4)=2/(-2)=-1#

Slope of the curve at point #(-2,1)# is the slope of the tangent.

Slope of the tangent is #m=-1#

enter image source here