Question

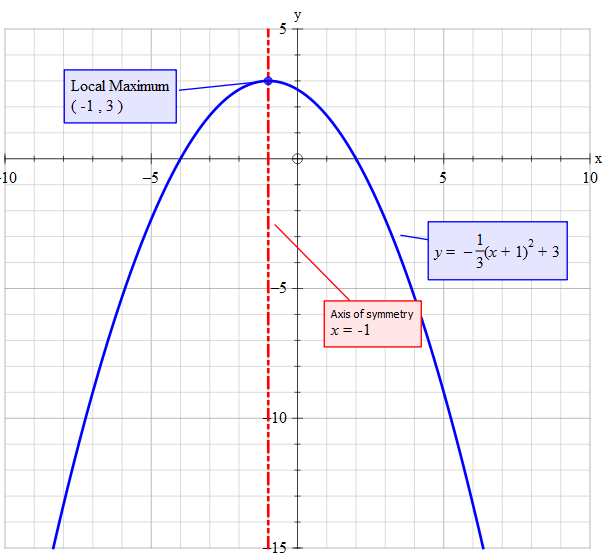
How do you graph the quadratic function and identify the vertex and axis of symmetry for `y=-1/3(x+1)^2+3`?

Answer 1

Vertex`->(x,y)=(-1,3)`

Axis of symmetry is at `x=-1`

Explanation:

If you were to expand the brackets you would get: `y=-1/3x^2.....`
As the coefficient of `x^2` is negative the graph is of form `nn`

The given equation is the vertex form of a quadratic. It is called that as you may read off the vertex coordinates with only a little but of adjustment.

Given:`" "y=-1/3(xcolor(red)(+1))^2color(blue)(+3)`

`x_("vertex")=(-1)color(red)(xx(+1) =-1`
`y_("vertex")=color(blue)(+3)`

Vertex`->(x,y)=(-1,3)`

Axis of symmetry is at `x=-1`