Question

How do you solve `2sin^2x = 1 + cosx` for `0° <= x <= 180°`?

Answer 1

`x=60^@, or, x= 180^@`.

Explanation:

`2sin^2x=1+cosx`.

`:. 2(1-cos^2x)=1+cosx`.

`:. 2(1+cosx)(1-cosx)-(1+cosx)=0`.

`:. (1+cosx){2(1-cosx)-1}=0`.

`:. (1+cosx)(1-2cosx)=0`.

`:. cosx=-1, or, cosx=1/2`.

`cosx=-1, 0^@<=x<=180^@"gives "x=180^@, and,`

`cosx=1/2" with "x" in the given range gives "x=60^@`.

Answer 2

The solutions are `x=60^@,180^@`.

Explanation:

You can use this altered Pythagorean identity:

`sin^2=1-cos^2x`

Now here's the actual problem. The strategy is to get everything in terms of `cosx`, then factor it like a quadratic. Here's what that looks like:

`2sin^2x=1+cosx`

`2(1-cos^2x)=1+cosx`

`2-2cos^2x=1+cosx`

`-2cos^2x+2=1+cosx`

`-2cos^2x-cosx+2=1`

`-2cos^2x-cosx+1=0`

`2cos^2x+cosx-1=0`

`2cos^2x+2cosx-cosx-1=0`

`color(red)(2cosx)(cosx+1)-cosx-1=0`

`color(red)(2cosx)(cosx+1)-color(blue)1(cosx+1)=0`

`(color(red)(2cosx)-color(blue)1)(cosx+1)=0`

`cosx=1/2,cosx=-1`

Here's a unit circle to remind us of some cosine values:

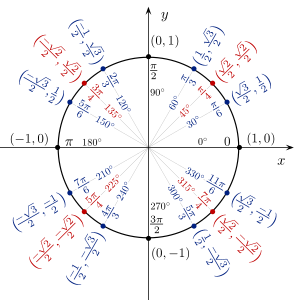
This means that:

`x=60^@,color(red)cancelcolor(black)(300^@),180^@`
`qquadqquad qquadqquad quad uarr`
`"not in the desired interval"`

Therefore, the solutions are `x=60^@,180^@`.

Answer 3

`x=60^@, or, x=180^@`.

Explanation:

`2sin^2x=1+cosx`.

`:. (1-2sin^2x)+cosx=0`.

`:. cos2x+cosx=0`.

`:. 2cos((2x+x)/2)cos((2x-x)/2)=0`.

`:. 2cos(3/2x)cos(1/2x)=0`.

Since, `0^@<=x<=180^@, 0^@<=1/2x<=90^@, and,`

`0^@<=3/2x <= 270^@`.

`:. cos(3/2x)=0 rArr 3/2x=90^@, 270^@`.

`rArr x=60^@, 180^@`.

`cos(1/2x)=0 rArr 1/2x=90^@, or, x=180^@`.

Altogether, `x=60^@, or, x=180^@`, as Before!