How do you solve for x in the given problem using right triangle trigonometry?

1 Answer
Apr 10, 2018
Explanation:
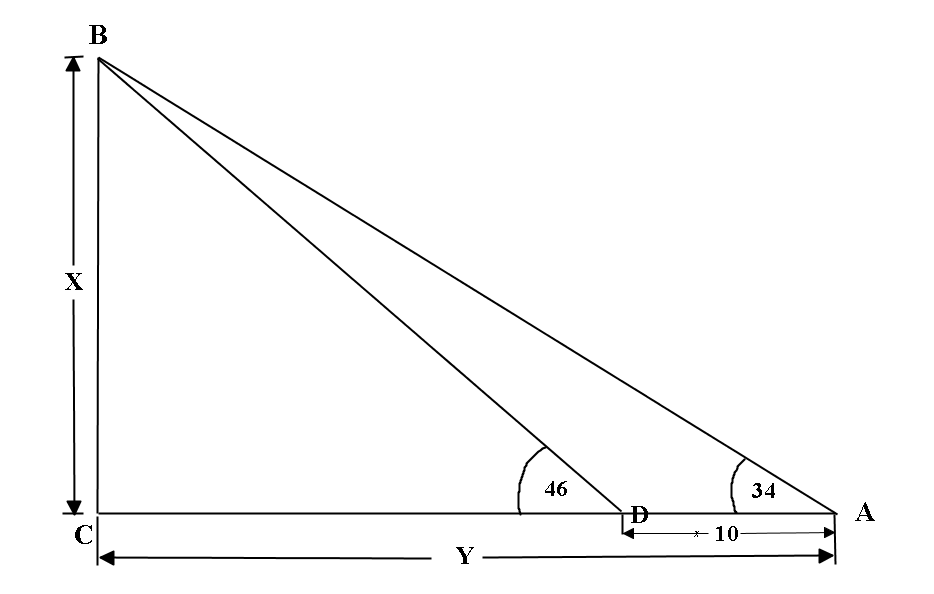
Using the tangent function:
Triangle ABC
Triangle DBC
Substituting in


Using the tangent function:
Triangle ABC
Triangle DBC
Substituting in