How do you solve #\frac { 1} { c + 10} = \frac { c } { 26}#?
2 Answers
Explanation:
Bring everything to the
Least common denominator is
A fraction equals zero only if its numerator equals zero:
Quadratic formula to solve:
So, the two solutions are
A slightly different beginning.
Explanation:
Given:
I wish to have the
Multiply both sides by c
Subtract 26 from both sides
You will not have whole number factors so use the formula.
In its normally remembered form we have:
You are looking for squared factors that you can 'take outside' the root.
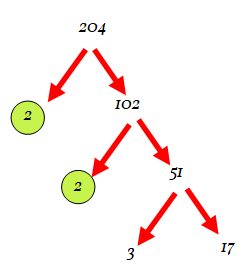
If you are ever uncertain about factoring larger values draw a quick sketch of a prime factor tree.