How do you use Newton's method to find the approximate solution to the equation #x^4=x+1,x<0#?

Question

2800 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-12-29T21:53:25

#x=-0.72449# to 5dp

We have:

# x^4=x+1 => x^4-x-1 = 0 #

Let #f(x) = x^4-x-1# Then our aim is to solve #f(x)=0#

First let us look at the graph:
graph{x^4-x-1 [-3, 3, -5, 8]}

We can see there is one solution in the interval # -1 < x < 0 # and a solution in # 1 < x < 2 #

In order to find the solution numerically, using Newton-Rhapson method, we need the derivative #f'(x)#

# f(x) = x^4-x-1 => f'(x) = 4x^3-1 #,

and the Newton-Rhapson method uses the following iterative sequence

# { (x_0,=0), ( x_(n+1), = x_n - f(x_n)/(f'(x_n)) ) :} #

Then using excel working to 5dp we can tabulate the iterations as follows:

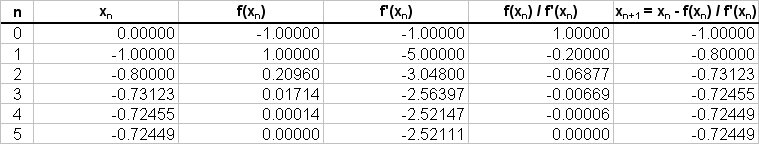
enter image source here

And we conclude that a solution is #x=-0.72449# to 5dp