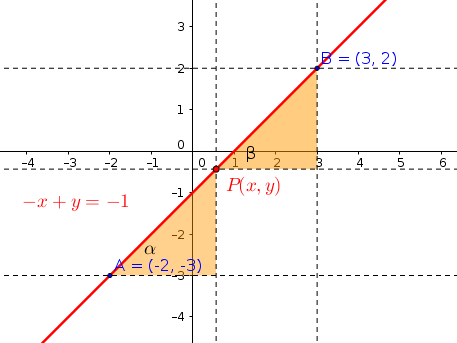
"let P(x,y) be a Point on the line passed A(-2,-3) and B(3,2)."let P(x,y) be a Point on the line passed A(-2,-3) and B(3,2).
"Since "alpha=beta ," we can write as "tan alpha=tan betasince α=β, we can write as tanα=tanβ
tan alpha=(y+3)/(x+2)tanα=y+3x+2
tan beta=(2-y)/(3-x)tanβ=2−y3−x
(y+3)/(x+2)=(2-y)/(3-x)y+3x+2=2−y3−x
(y+3)(3-x)=(x+2)(2-y)(y+3)(3−x)=(x+2)(2−y)
3y-xy+9-3x=2x-xy+4-2y3y−xy+9−3x=2x−xy+4−2y
3y+2y-cancel(x y)+cancel(x y)-3x-2x=+4-9
cancel(5)y-cancel(5)x=-cancel(5)
y-x=-1
-x+y=-1

