Question

How many solutions does `sin^2(2x)=1` have for `0≤(x)≤(3π)`?

(Without using a graphing calculator)

Answer 1

The equation has `6` solutions on the given interval:

`pi/4,(3pi)/4,(5pi)/4,(7pi)/4,(9pi)/4,` and `(11pi)/4`.

Explanation:

`sin^2(2x)=1`

`sqrt(sin^2(2x))=+-sqrt1`

`sin(2x)=+-1`

Here's a unit circle to remind us of some `sin` values:

`2x=pi/2,(3pi)/2,(5pi)/2,(7pi)/2,(9pi)/2, (11pi)/2...`

`x=(pi/2)/2,((3pi)/2)/2,((5pi)/2)/2,((7pi)/2)/2,((9pi)/2)/2,((11pi)/2)/2...`

`x=pi/4,(3pi)/4,(5pi)/4,(7pi)/4,(9pi)/4, (11pi)/4...`

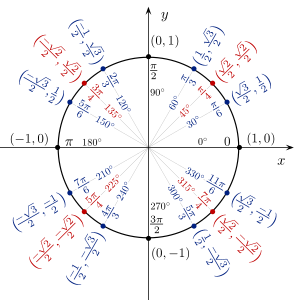
These are the only solutions in the given range `[0,3pi)`. There are six solutions, so that is the answer.