How much work is required for an astronaut to lift the Mars Rover with a mass of 2300 kg on the moon?
1 Answer
A force greater than 3730.6N is required to move the object.
Explanation:
To begin, work is essentially a fork applied over a distance. If the force isn't applied in the direction of the motion, it is said to not contribute to the work being applied on the object.
A distance wasn't stated, so I'm assuming that you're in search of the force required to disturb the equilibrium - meaning that the Mars Rover, positioned on the moon, is in a state of equilibrium as long as there is no motion. Once an external force is applied, the state is broken and the problem then becomes dynamics rather than statics. As soon as you know the force required, you can apply the linear work equation to solve for the work required at any distance.
To properly find out the force required to break equilibrium, you must consider all of the factors of the problem: the weight of the rover, the gravity of the moon... can you think of any others? Always start a mechanics problem with a free body diagram - it will make everything a lot easier later on, in more complex situations!
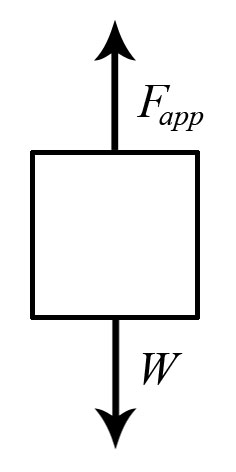
Once you have a FBD set up, you can determine what each equation is, and what you need to solve for each.
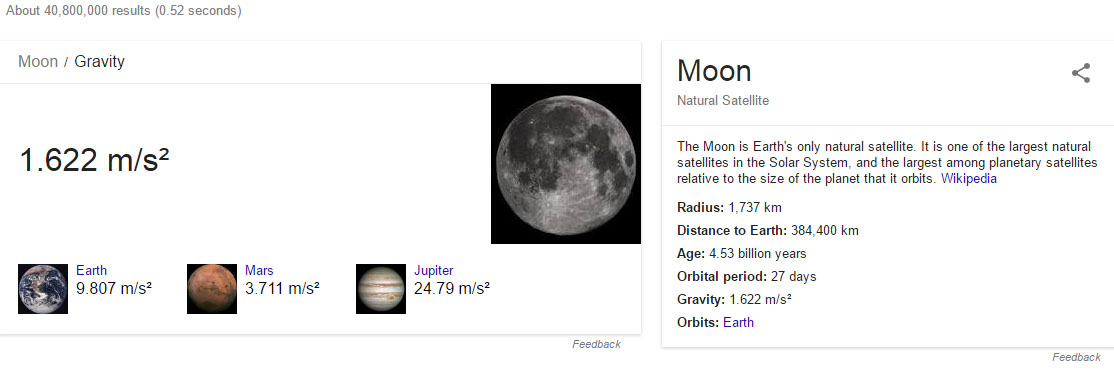
Through your textbooks or online resources, such as Google, you can determine the constants required to help you accurately solve the problem.
Taking 1.622
Weight (
Since the forces only act in the vertical direction, and there are no other forces acting upon the Rover, the force that must be applied to maintain the equilibrium must be equal in magnitude, but opposite in direction. In order to break the equilibrium, the force applied must be greater than the weight of the object while acting in the opposite direction.
Thus, the solution should be a value greater than
Note: the formula for work in a linear motion:

