Question

How to answer these question using differentiation ?

The answer is `3/(4pi) cm (min)^-1`

Answer 1

See the explanation: using 'older notation'

Explanation:

Let the radius of the water surface at any point be `r`
Let the height from the vertex to the water surface be `h`
Let the time in minutes be `t`

Volume of the water is `1/3pir^2h ->(pir^2h)/3`

We are given that the volume of water discharged into the cone is 3cm per minute

Given that there is a relationship to the height and time we have

`3t=(pir^2h)/3`

However, the shape of the cone is such that `r=h` so substituting for `r` we have

`3t=(pih^3)/3`

Divide both sides by 3

`t=(pih^3)/9`

`(dt)/(dh)=pi/9xx3h^2 = (pih^2)/3`

But to answer the question we need `(dh)/(dt)` so turn everything upside down (invert).

`(dh)/(dt)=3/(pih^2)`

At `h=2` we have `(dh)/(dt)=3/(4pi)color(white)(..) (cm^3)/("min")`

Answer 2

The required rate is `3/(4pi) ~~ 0.2387 cm^3 mi n^(-1)`

Explanation:

Let us set up the following variables:

# { (t, "time", min), (r, "radius of the top of the water ate time " t, cm), (h, "height of the water at time "t, cm), (V, "Volume of the water at time "t, cm^3) :} #

The volume of the cone is given by the standard formula:

`V = 1/3pir^2h`

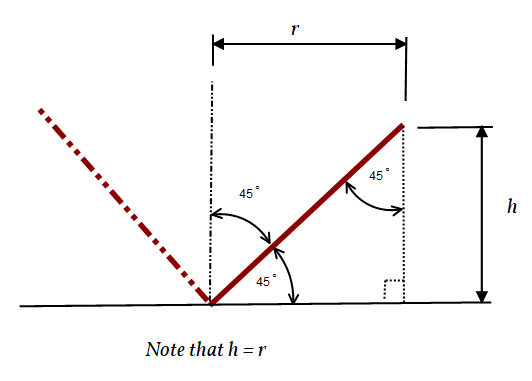
As the angle between the vertical and the slant is `45^o` then so is the angle between the base and the slant and so we have `r=h`, So:

`V = 1/3pih^2h`

`:. V = 1/3pih^3`

If we differentiate wrt '`t`and apply the chain rule., then:

`(dV)/(dt) = d/dt {1/3pih^3}`

`\ \ \ \ \ \ = 1/3pi \ d/(dh) {h^3} \ (dh)/dt`

`\ \ \ \ \ \ = 1/3pi \ 3h^2 \ (dh)/dt`

`\ \ \ \ \ \ = pi h^2 \ (dh)/dt`

We are given that "Water drop into the container ate a rate of `3cm^3` per minute", which tells us that:

`(dV)/(dt) = 3 \ \`, a constant

And so we have:

`pi h^2 \ (dh)/dt =3`

`:. (dh)/dt =3/(pi h^2)`

The question asks us to calculate "the rate at which the water level is increasing when the water level is `2cm`", so we require the value of `(dh)/dt` when `h=2`, which we get from:

`[ (dh)/dt]_(h=2) = 3/(pi 2^2)`

`\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ = 3/(4pi)`

`\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ ~~ 0.2387 \ cm^3 mi n^(-1)`