If a projectile is shot at a velocity of #12 m/s# and an angle of #pi/6#, how far will the projectile travel before landing?
1 Answer
The projectile has a range of about
Explanation:
We can find the projectile's total horizontal displacement using kinematics. We will first make the assumption that air resistance and all other outside forces are negligible and consider only the effects of the force of gravity.
We have the following information:
-
#theta=pi/6# -
#v_i=12" m"//"s"# -
#a_y=-g=-9.81" m"//"s"^2# -
#a_x=0" m"//"s"^2#
My approach would be to calculate the time elapsed over the motion using the vertical components and use this to find the horizontal displacement.
We will use the following kinematic equation and solve for
#color(blue)((v_f)_y=(v_i)_y+a_yDeltat)#
using the fact that at the top of the object's motion,
Before we can use this equation, however, we will have to find
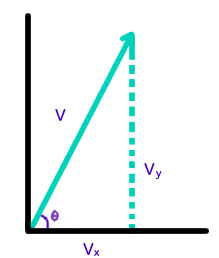
- This diagram represents only one velocity vector, that is, the first (
#v_i# ).
#sin(theta)="opposite"/"hypotenuse"#
#=>sin(theta)=v_y/v#
#=>color(blue)(v_y=vsin(theta))#
#=>=12" m"//"s"*sin(pi/6)#
#=>=(12)/2#
#=>=color(blue)(6" m"//"s")#
We can now make use of the above kinematic equation and solve for the flight time from laugh to maximum altitude.
#(v_f)_y=(v_i)_y+a_yDeltat#
#=>Deltat=(cancel(v_f)-v_i)/a#
#=(-6" m"//"s")/(-9.81" m"//"s"^2)#
#~~color(blue)(0.612"s")#
Assuming the launch and landing altitudes are equal (as we are not told otherwise), the total flight time is twice the rise time, or
We can now put this time into a new equation to find
#Deltax=(v_i)_xDeltat+cancel(1/2a_xDeltat^2)#
#Deltax=(v_i)_xDeltat#
As above, we find the parallel component of the initial velocity using trigonometry. We get
#=(12"m"//"s")cos(pi/6)(1.22" s")#
#~~12.7"m"#
#=>color(blue)(13"m")#