In what direction should you swim across a river if you want to minimize the time spent in the water?
2 Answers
Perpendicular to the edge of the river
Explanation:
By this way, the distance will be minimized so it becomes the smallest possible distance traveled (essentially it is the displacement), and if the distance is minimized the time will be minimized according to the equation
Where 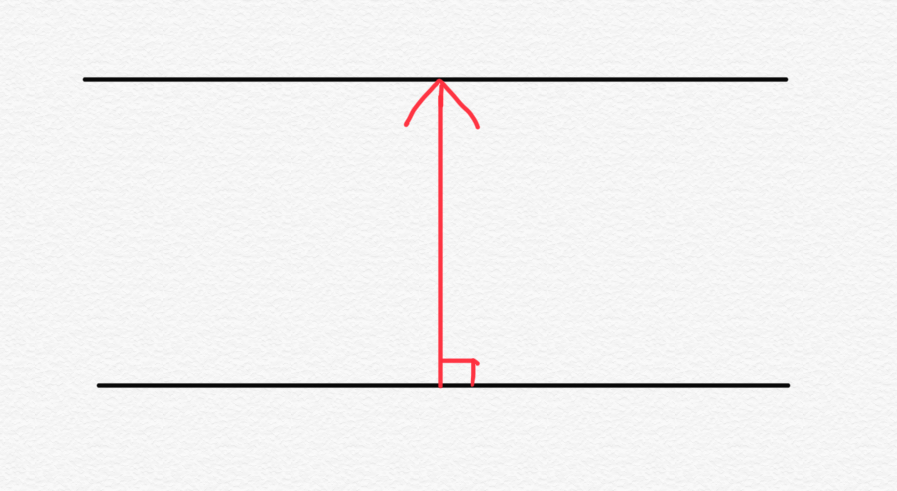
See explanation...
Explanation:
Seek to swim perpendicular to the bank, but do not adjust to compensate for the water carrying you downstream.
That way, your swimming is not wasted in fighting against the current, but all the motion contributed by your swimming will be effective.
If the width of the river is
So if you wanted a point to aim for on the opposite bank, then you could aim for a point at angle
If you instead attempted to swim the shortest distance across the river, then you would actually be in the water somewhat longer.
One way of thinking about this problem is to consider the frame of reference of the river, rather than the land.