Question

Is it possible for a line to have no x-intercept and no y-intercept?

Answer 1

Yes

Explanation:

The line passing through the origin: `y=mx` has both x-intercept & y-intercept zero in XY-plane

Answer 2

No.

Explanation:

A line can have no x-intercept or no y-intercept, but not both.

The x-intercept is where a line crosses the x-axis. The y-axis is where a line crosses the y-axis.

The equation of a vertical line is `x=h`. A vertical line crosses the x-axis, but not the y-axis, as long as `x!=0`. For example, a vertical line such as `x=-4` has an x-intercept of `(-4,0)`, but there is no y-intercept because the line does not cross the y-axis.

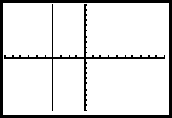
The equation of a horizontal line is `y=k`. A horizontal line crosses the y-axis, but not the x-axis, as long as `y!=0`. For example, a horizontal line such as `y=-3` has a y-intercept of `(0,-3)`, but there is no x-intercept because the line does not cross the x-axis.

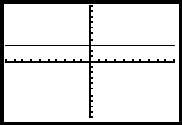
http://algebralab.org/lessons/lesson.aspx?file=Algebra_LinearEqIntercepts.xml