Period of conical pendulum?
1 Answer
Feb 20, 2018
See the explanation below
Explanation:
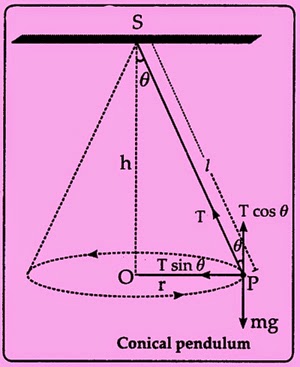
The period of a conical pendulum is
See the explanation below

The period of a conical pendulum is