Question

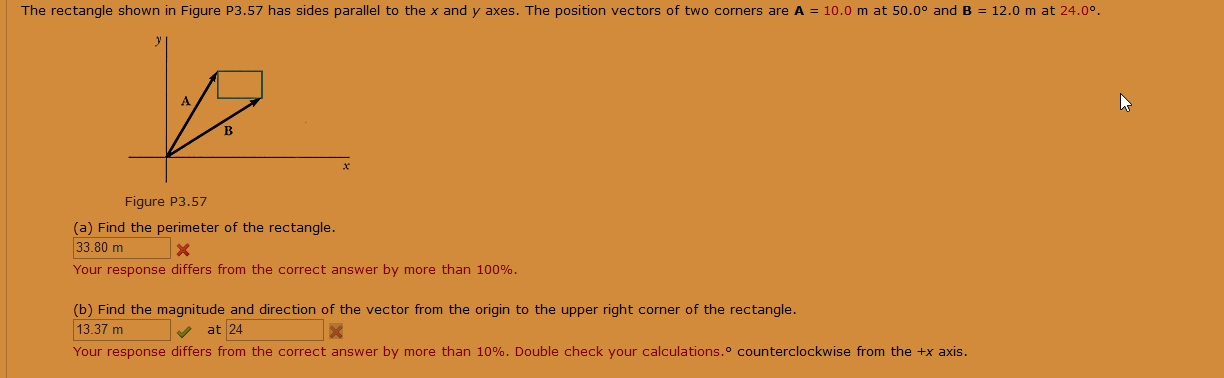
Physics Question?The rectangle shown in Figure P3.57 has sides parallel to the x and y axes. The position vectors of two corners are A = 10.0 m at 50.0° and B = 12.0 m at 24.0°.

Answer 1

Perimeter = `2 = 14.62`
`C = 13.4`
`phi = 34.9`

Explanation:

Whether using vectors or diagrams, it is essentially a geometry problem. The two trianglyes defined by the angles and the given sides (vectors) with the x-axis can be solved for the rectangle side lengths.

The "A" triangle has a hypotenuse of 10 and an angle of 50, from which we calculate `x_1/10 = cos(50)` and `y_1/10 = sin(50)`

The "B" triangle has a hypotenuse of 12 and an angle of 24, from which we calculate `x_2/12 = cos(24)` and `y_2/12 = sin(24)`_2

The sides are then `x_1 - x_2` and `y_1 - y_2`.

`x_1 = 6.43` ; `y_1 = 7.66`
`x_2 = 10.96` ; `y_2 = 4.88`

`x_1 - x_2 = 4.53` and `y_1 - y_2 = 02.78`.
Perimeter = `2xx(4.53 + 2.78) = 14.62`

To find the length and angle of the vector to the far corner we construct another triangle with Hypotenuse C, height `y_1` and base of `x_2`.
`C^2 = x_2^2 + y_1^2` ; `C^2 = 120.1 + 58.7`
`C = 13.4`
The angle is thus `tan(phi) = y_1/x_2 = 7.66/10.96 = 0.7`
`phi = 34.9`