Question

`R = 7/(4 − 6 sin(theta))` Convert the polar equation to rectangular form. ?

Answer 1

I shall begin by graphing the original polar equation `r = 7/(4 − 6 sin(theta))`

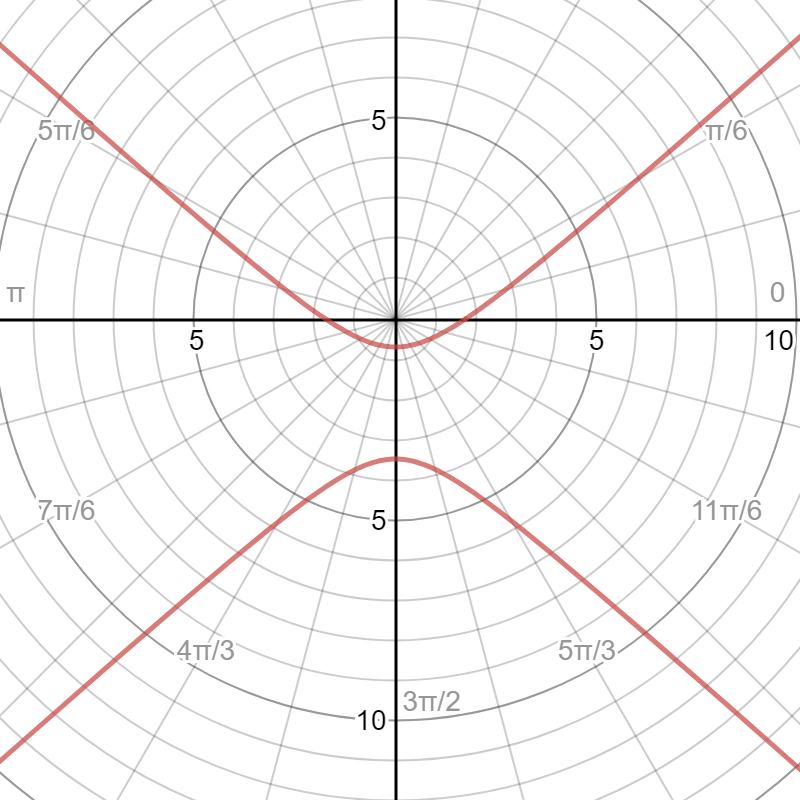
Multiply both sides by `4-sin(theta)`

`4r - 6rsin(theta) = 7`

Substitute `r = sqrt(x^2+y^2)` and `rsin(theta) = y`:

4sqrt(x^2+y^2) -6y = 7

Add `6y` to both sides:

4sqrt(x^2+y^2) = 6y + 7

Square both sides:

`16(x^2+y^2) = (6y+7)^2`

Expand the squares:

`16x^2+16y^2 = 36y^2+84y+49`

This is the standard Cartesian form for conic section:

`16x^2-20y^2 -84y- 49 = 0`

Here is its graph:

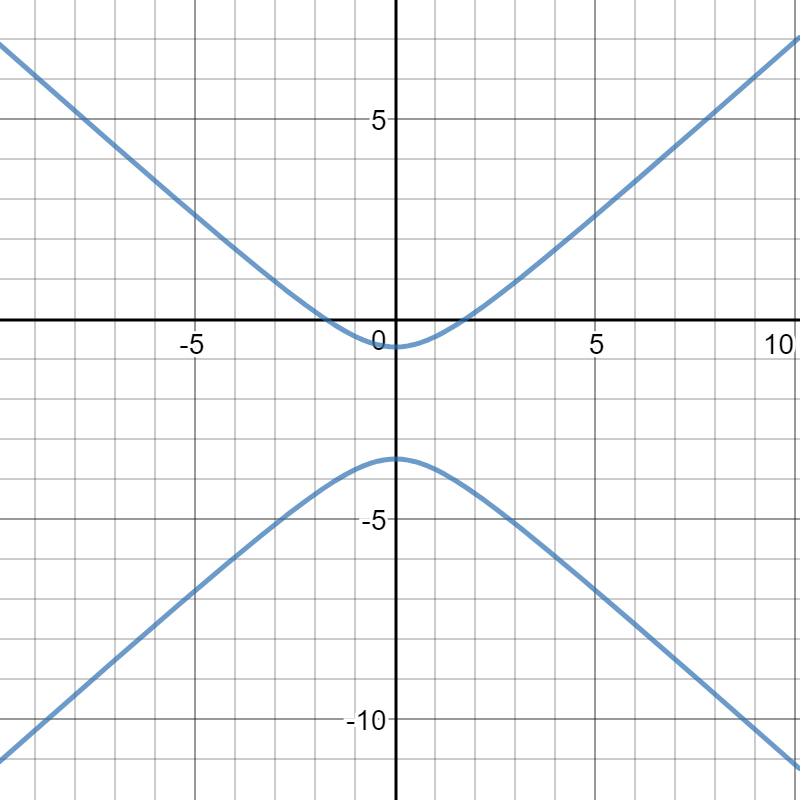
Please observe that the two graphs are identical.