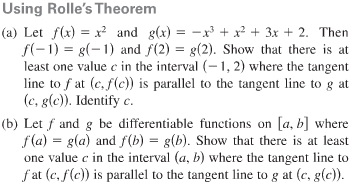
To show that the tangent line to the graph of f at (c,f(c)) is parallel to the tangent line to the graph of g at (c,g(c)), it suffices to show that f'(c) = g'(c).
Let D be the difference f-g
So D(x) = f(x) - g(x)
Observe that
D'(x) = f'(x)-g'(x), so D is differentiable and therefore continuous on [-1,2]. (Part (b), "on [a,b].")
Furthermore, D(-1) = 0 and D(2) = 0 (Part (b) substitute a and b.)
By Rolle's Theorem, there is a c in (-1,2) (in [a,b]) such that
D'(c) = 0
The implies that f'(c) - g'(c)=0 so f'(c) = g'(c).
To find the c in part (a), find D'(x), set it to 0 and solve in the interval (-1,2).
D(x) = (x^2)-(-x^3+x^2+3x+2) = x^3-3x-2
So D'(x) = 3x^2-3
and 3x^2-3 = 0 at x = +-1.
-1 is not in (-1,2) but 1 is
so the only possible value for c is c = 1.