Solve this?
From the foot of a column the angle of elevation of the top of a tower is 45° and from the top of column the angle of depression of the bottom of the tower is 30°. A man walks 10 meters from the bottom of the column towards the tower. He notices the angle of elevation of its top to be 60°. Find the heights of the column and the tower.
From the foot of a column the angle of elevation of the top of a tower is 45° and from the top of column the angle of depression of the bottom of the tower is 30°. A man walks 10 meters from the bottom of the column towards the tower. He notices the angle of elevation of its top to be 60°. Find the heights of the column and the tower.
1 Answer
Apr 30, 2018
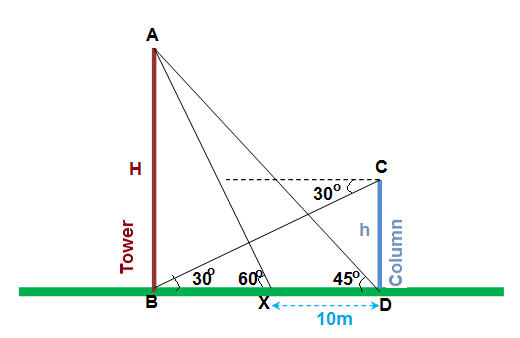
In
So the triangle is right isosceles
and
Now
Now

