Question

The sum of the measures of the interior angles of a convex polygon is 3060°. How do you classify the polygon by the number of sides?

Answer 1

This is a `19`-sided polygon, called an enneadecagon or nonadecagon.

Explanation:

`3060^@ = 17 xx 180^@`

The internal angles of a (plane) triangle have sum `180^@`

So (at least if it is convex) this polygon can be divided into `17` triangles using non-intersecting straight line cuts through pairs of vertices.

That means it has `19` sides.

Answer 2

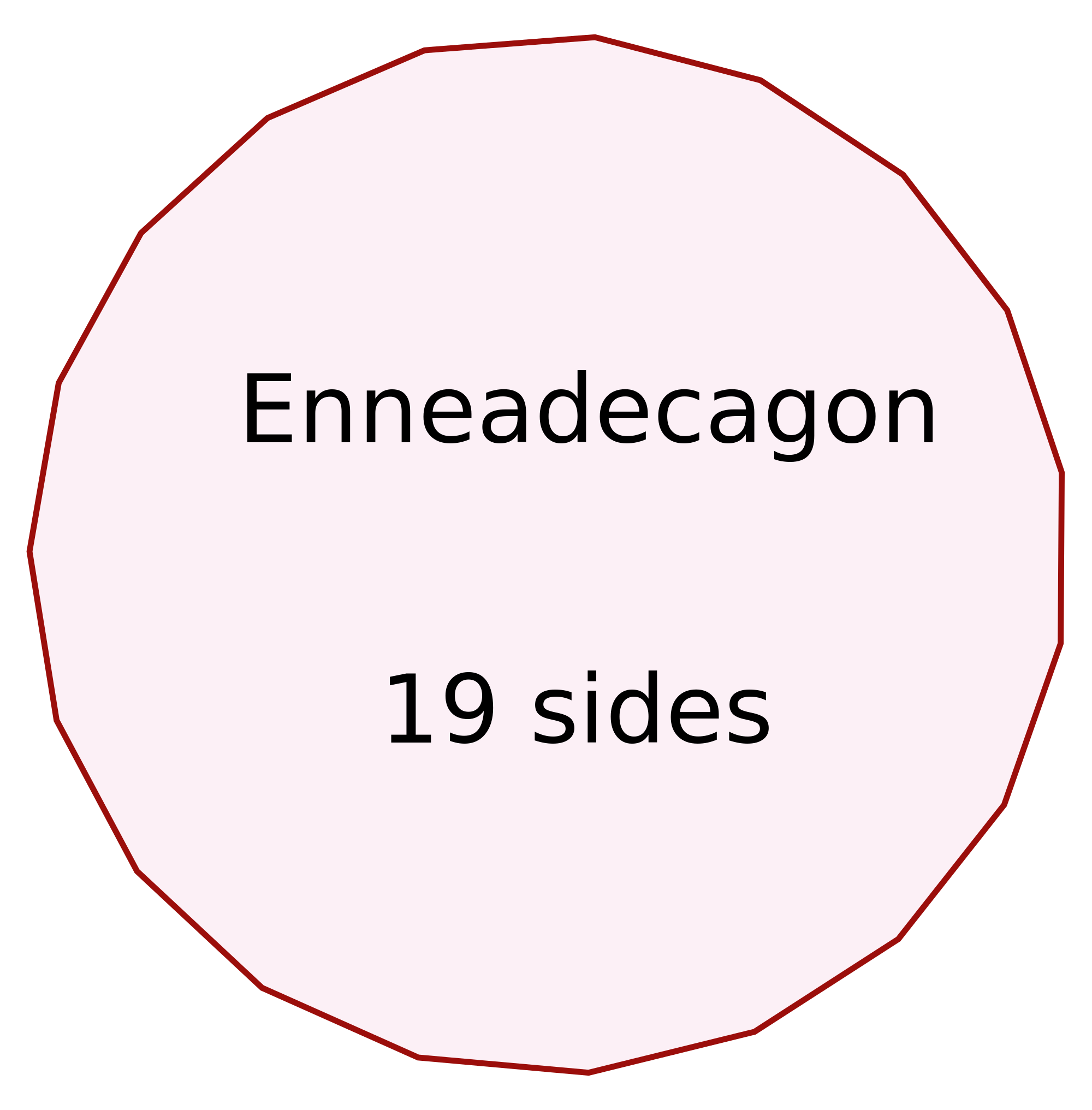
A nonadecagon or an enneadecagon with `19` sides

Explanation:

Always remember the formula (very important)

The sum of the interior angles of a convex polygon is

`color(indigo)((n-2)180`

Where `n` is the number of sides.

Now get straight into the question

They have given that the sum of the interior angles of the polygon is `3060^circ`

So,

`rarr(n-2)180=3060`

Divide both sides by `180`

`rarr((n-2)cancel180)/cancel180=3060/180`

`rarrn-2=17`

Add `2` both sides

`rarrn-2+2=17+2`

`color(green)(rArrn=19`

This is the polygon with `19` sides

source of image: Wikipedia