Two buildings are 80 feet apart. From the roof of the taller building, which is 300 feet high, the angle of depression to the edge of the shorter building is 30◦ What is the height of the shorter building?

1 Answer
Somebody N.
Feb 27, 2018

#253.81color(white)(888)#feet

Explanation:

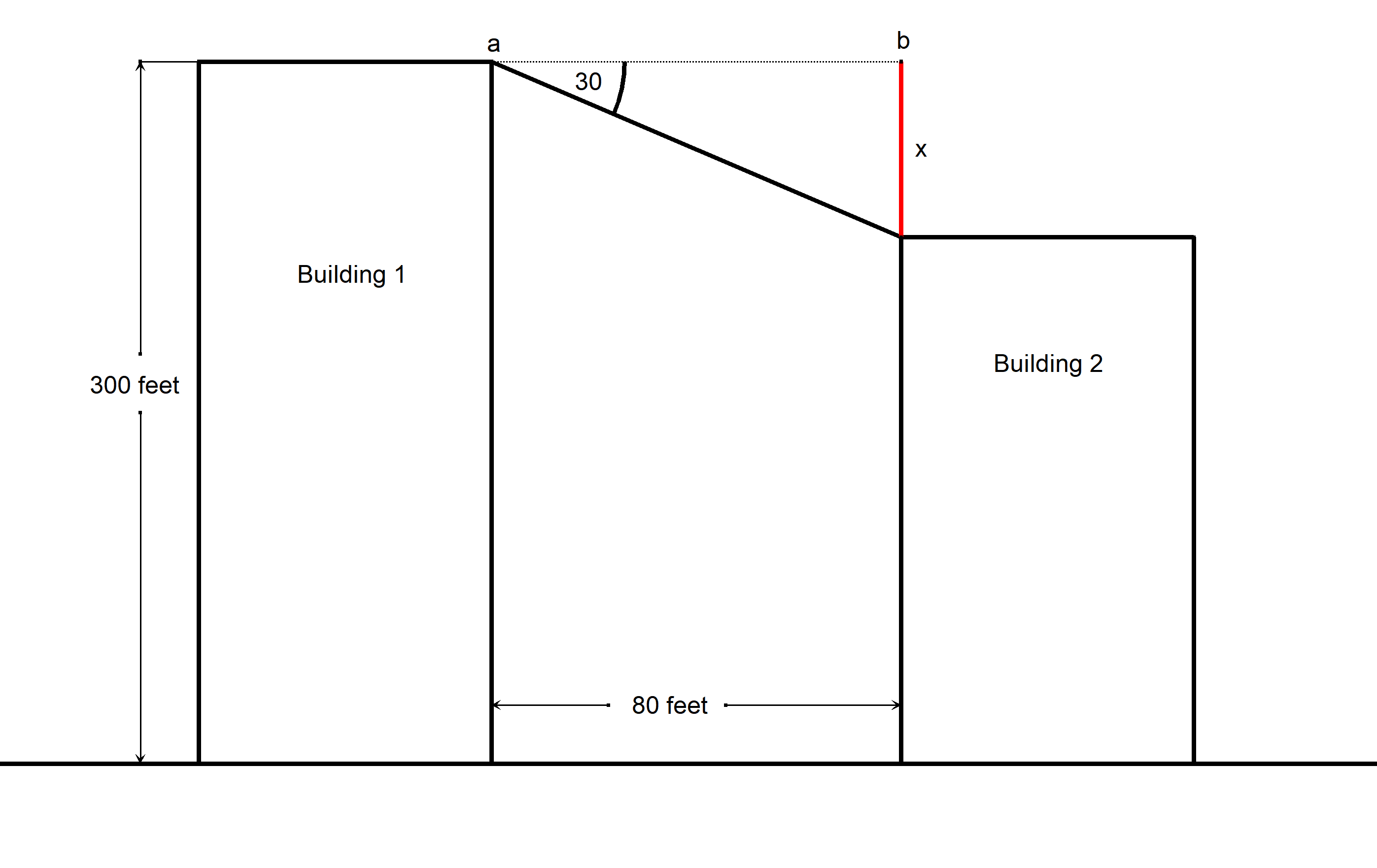
enter image source here
From the diagram. If we calculate the length of #bbx# shown in red, this will give us the difference in height of the two buildings. We can then subtract this difference from the taller building to find the height of the smaller building.

We can use the #color(blue)("tangent function")# to solve this.

The length of the line ab is equal to the distance between the buildings.

.i.e. #bb("80 feet")#.

#tan(theta)="opposite"/"adjacent"#

In our case this will be:

#tan(30)=x/(ab)=x/30#

We need to find #x#.

Rearranging:

#x=80tan(30)#

#x=46.19color(white)(88888)# ( 2 d.p.)

Subtracting this from the height of the taller building:

#300-46.19=253.81color(white)(888)#feet

Related questions
Impact of this question
1824 views around the world
You can reuse this answer
Creative Commons License