Using the integral test, how do you show whether sum 1 / [sqrt(n) * (sqrt(n) + 1)] diverges or converges from n=1 to infinity?
1 Answer
Jun 13, 2016
The sum is unbounded
Explanation:
We know
because
but
so the sum is unbounded
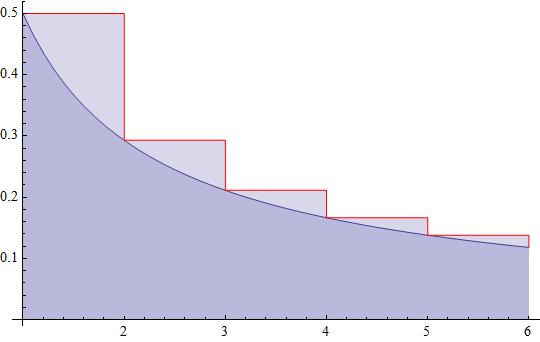
Attached a figure with the comparisson between series and integral.