Question

What happens to the speed of a wave if the wavelength decreases and frequency stays the same?

Answer 1

Wavelength is the horizontal distance from one part of the wave to the very next spot on the wave that is indistinguishable from the current spot.

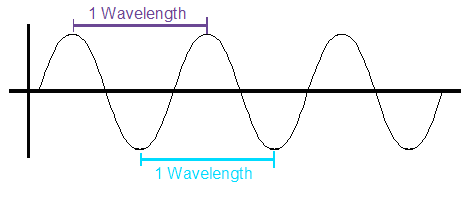
In the above picture, you can see that the wavelength can go from one crest to another crest or one trough to another trough.

Frequency is how often a specific point (and all indistinguishable points) on a wave travels, over time, past a user-specified spot in space, which is why its units are `"cycles/s"` ("cycles per second").

The above wave shows 3 cycles. So, if the entire above wave had just traveled past the y-axis in 3 seconds, we would say that it traveled `"3 cycles/3 s"` or `"1 cycle/s"`.

If we suppose wavelength decreases but frequency remains constant (like when you enter a different medium with monochromatic light; the light color doesn't change), the speed of the wave changes according to the following equation:

`\mathbf(v = lambdanu)`

where:

• `v` is speed (it's the english letter "v")
• `nu` is frequency (it's the greek letter "nu")
• `lambda` is wavelength

So, when `lambda` decreases, `v` decreases under the assumption that `Deltanu = 0`.

For example, that would say that colored light shooting through water moves more slowly even though it's the same frequency, because its wavelength is shorter in that thicker medium relative to air (the index of refraction of water is about `1.33`, while that of air is almost `1.0`).