What is the difference between a definite and indefinite integral?
1 Answer
A definite integral has limits of integration and the answer is a specific area. An indefinite integral returns a function of the independent variable(s).
Explanation:
A definite integral has limits of integration, for example:
where
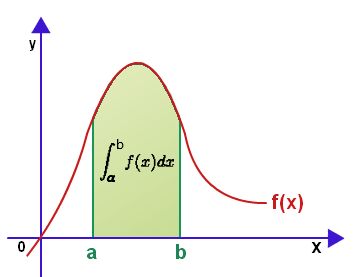
Most of the time, we solve the integral to get a new function of
Where
An indefinite integral has no limits specified:
and so returns a function of the independent variable(s) plus an arbitrary constant. In this case it is easy to see that when we take the derivative of both sides, the constant disappears, which is why it is a possible part of the solution of the integral in the first place.
ASIDE:
Sometimes the indefinite integral is not possible to find in a closed form (or without special functions that only have approximate forms), but definite integrals with specific limits are possible to find through various tricks. For example, the total area under the bell curve
Where we can't get a simple solution in terms of

